nForum
Not signed in
Want to take part in these discussions? Sign in if you have an account, or apply for one below
Site Tag Cloud
Vanilla 1.1.10 is a product of Lussumo. More Information: Documentation, Community Support.
If you want to take part in these discussions either sign in now (if you have an account), apply for one now (if you don't).
-
- CommentRowNumber1.
- CommentAuthorEric
- CommentTimeApr 10th 2010
I asked a question about field in this discussion, but I’m obviously confused, so I thought I’d take a step backward and ask about abelian groups here.
Is there a nice “arrow theoretic” way to define an abelian group?
A group (or maybe I should say a delooping of a group?) is a category with one object and all morphisms are invertible. All the group axioms follow from this.
The morphisms are the elements of the group and composition is the group operation.
Stating does NOT seem to be very natural or “arrow theoretic”.
So while noncommutative groups are perfectly naturally defined via arrows, a commutative group requires something put in by hand.
As I mentioned in this comment, commutativity often arises from noncommutativity (in my experience) in some kind of “continuum limit”.
So I offered a speculative suggestion that translates (sort of) to abelian groups.
Speculation: An abelian group is a group whose elements are close to 1.
One way I can think of to try to put some calculus into it is to maybe say that an element is “close to 1” if it can written as
where is an element of some ring and
I don’t know if this buys us anything though…
-
- CommentRowNumber2.
- CommentAuthorDavidRoberts
- CommentTimeApr 10th 2010
- (edited Apr 10th 2010)
An abelian group 'is' a 2-groupoid with only one object, and one 1-arrow. The Eckmann-Hilton argument tells you that the two operations (vertical and horizontal composition), because they share the same identity element, are equal (so you really only have one operation), and that it is abelian. This is precisely why
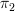 is abelian, because it is secretly just a 2-groupoid without the 0- or 1-dimensional information.
is abelian, because it is secretly just a 2-groupoid without the 0- or 1-dimensional information.Also, any
 -groupoid with only trivial
-groupoid with only trivial 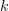 -arrows up to
-arrows up to  -arrows is basically just an abelian group.
-arrows is basically just an abelian group.The trick you were trying to pull in the other thread won't work, as the 'addition' and 'multiplication' degenerate into the same operation. Note that the category of fields isn't algebraic, because of the condition
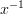 exists for all
exists for all 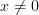 , and so I don't think there is a way of describing fields as a groupoid or higher groupoid, categories of which are (semi-)algebraic (I hope an expert drops in and supplies the 100% rigorous version of this statement)
, and so I don't think there is a way of describing fields as a groupoid or higher groupoid, categories of which are (semi-)algebraic (I hope an expert drops in and supplies the 100% rigorous version of this statement) -
- CommentRowNumber3.
- CommentAuthorUrs
- CommentTimeApr 10th 2010
I have expanded abelian group a little
-
- CommentRowNumber4.
- CommentAuthorDavidRoberts
- CommentTimeApr 10th 2010
that should be 2-truncated 1-connected at abelian group, but I can't edit the nlab at the moment.
-
- CommentRowNumber5.
- CommentAuthorUrs
- CommentTimeApr 10th 2010
that should be 2-truncated 1-connected
There might be two different conventions on how to count. I was following the one at connected, I think. But let me know if you think I am off. Like: off by one. :-)
-
- CommentRowNumber6.
- CommentAuthorDavidRoberts
- CommentTimeApr 10th 2010
- (edited Apr 10th 2010)
isn't 2-connected
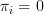 for
for  ? I need to change connected!
? I need to change connected!Edit: I mean, someone needs to change connected, and I can do it if it can wait until monday and work internet :P
-
- CommentRowNumber7.
- CommentAuthorzskoda
- CommentTimeApr 10th 2010
At least in traditonal homotopy theory, David is right. I do not know any authority for whom this is different. Usual connectedness is 0-connectedness, in particular.
-
- CommentRowNumber8.
- CommentAuthorDavidRoberts
- CommentTimeApr 10th 2010
Well, in shape theory I suppose it would be different, since then the Warsaw circle is simply connected (or 1-connected, depending on terminology) but has nontrivial shape in dimension one.
-
- CommentRowNumber9.
- CommentAuthorEric
- CommentTimeApr 10th 2010
Thank you David and thank you Urs.
Note that the category of fields isn’t algebraic, because of the condition x^{-1} exists for all x \ne 0, and so I don’t think there is a way of describing fields as a groupoid or higher groupoid, categories of which are (semi-)algebraic (I hope an expert drops in and supplies the 100% rigorous version of this statement)
Me too. Fields have always bothered me more than abelian groups, so I’m happy to see a nice (beautiful even!) arrow theoretic description of abelian groups. I’m sure I’ve seen that before, but I’m only now trying to spend some time on this stuff after years of only peripheral attention (including careers changes, babies, moving several times, etc).
What are the implications for a category to not be algebraic? Does that mean it cannot be described via arrow theory?
-
- CommentRowNumber10.
- CommentAuthorMike Shulman
- CommentTimeApr 10th 2010
I’ve always thought that connected = 0-connected, and I went ahead and changed the page connected appropriately. As Zoran says this has been the usage in ordinary homotopy theory for a long time, so I really don’t think we should try to change the meaning in category theory. Of course, this means that there is another case when a “thing” is not the same as a “1-thing,” but I think we can deal. Note that HTT sidesteps this issue by saying “n-connective” to mean what a homotopy theorist would call “(n-1)-connected”.
-
- CommentRowNumber11.
- CommentAuthorMike Shulman
- CommentTimeApr 10th 2010
What are the implications for a category to not be algebraic? Does that mean it cannot be described via arrow theory?
I don’t know what “arrow theory” means, so I can’t answer that, but not-algebraic categories can still of course be studied using category theory. The category of fields does have some nice properties, for instance it is finitely accessible.
-
- CommentRowNumber12.
- CommentAuthorDavidRoberts
- CommentTimeApr 10th 2010
My only conclusion (sketchy though it may be) is that the category of (strict!) n-categories is as far as I understand it, a semi-algebraic theory (it's very late here, so forgive me if this is a bit muddled), but the category of fields isn't algebraic, so it is unlikely that fields can be described as categories such that... in a natural way. This logic seems sketchy to me too, but that is what I meant in the above post.
Of course, one can be silly and say that a field is, under addition, an abelian group, hence a 2-groupoid with a single object and a single 1-arrow, such that the set of 2-arrows is a ring (so there is a bilinear multiplication), and actually a field, but if I understand what you mean by arrow theory, this isn't sufficent. My guess is that you could get so far and then it will just turn into a repackaging of the usual definition of field using arrows instead of elements of a set.
Recently I came to realise that the next best thing to a field is a local ring - it's a ring which has the nice property that all non-invertible elements form an ideal (and this ideal has some special property I can't recall), and so you can quotient by the ideal and get a field (this isn't the defining property, see the page for details). In algebraic geometry and number theory local rings are all over the place. I don't know if an 'arrow theory' would work for local rings either, but the realisation was nice. Most important for my own particular tastes is the fact that local rings can be defined internal to (certain) categories, unlike fields.
-
- CommentRowNumber13.
- CommentAuthorHarry Gindi
- CommentTimeApr 10th 2010
- (edited Apr 10th 2010)
@Eric: Fields cannot be described arrow theoretically. The category of fields is one of the worst-behaved categories around. For example, all morphisms are monic, and there is no inital or terminal object. The category has binary pullbacks, and it has pushouts only when there exists a third field extension containing the two that we are pushing out. This is called the compositum of fields.
There may be a way to describe a field as a particular sort of object in the category of commutative rings, but almost certainly not purely arrow theoretically. -
- CommentRowNumber14.
- CommentAuthorDavidRoberts
- CommentTimeApr 10th 2010
There is a set of weakly initial objects, though - the prime fields, of which there are countably many. But may not be a particularly helpful fact given the question.
http://mathoverflow.net/questions/3003/in-what-sense-are-fields-an-algebraic-theory
for reference
-
- CommentRowNumber15.
- CommentAuthorHarry Gindi
- CommentTimeApr 10th 2010
- (edited Apr 10th 2010)
@David (response to your statement about fields): Quasilocal rings are rings with a unique maximal ideal. The beauty of quasilocal rings is that you can do linear algebra over them only being a little bit more careful than with fields. Even better than quasilocal rings are local rings, which are noetherian as well (i.e. all ideals are finitely generated). In fact, you can sharpen the theory more by considering discrete valuation rings, and so on. If you're interested, these notes from a course I'm currently taking are quite good. They describe techniques for working with arbitrary noetherian algebras over a field or a DVR by passing to nicer and nicer cases. Also, if you're interested in algebraic geometry, these notes go over a lot of the necessary stuff for étale cohomology.
With the stuff covered in these notes and a little bit of descent theory, you can develop a lot of algebraic geometry via the functor of points approach without ever constructing a scheme as a locally ringed space. This is very nice, because we can describe schemes and algebraic spaces simply as full subcategories of the topos of étale sheaves on
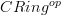 satisfying certain representability properties. Given your categorical background, this seems like it would be a very useful approach.
satisfying certain representability properties. Given your categorical background, this seems like it would be a very useful approach. -
- CommentRowNumber16.
- CommentAuthorUrs
- CommentTimeApr 11th 2010
Okay, thanks to David and Mike for fixing this. My fault.
1 to 16 of 16