nForum
Not signed in
Want to take part in these discussions? Sign in if you have an account, or apply for one below
Site Tag Cloud
Vanilla 1.1.10 is a product of Lussumo. More Information: Documentation, Community Support.
If you want to take part in these discussions either sign in now (if you have an account), apply for one now (if you don't).
-
- CommentRowNumber1.
- CommentAuthorUrs
- CommentTimeFeb 23rd 2010
added to shape theory a section on how strong shape equivalence of paracompact spaces is detected by oo-stacks on these spaces
By the way: I have a question on the secion titled "Abstract shape theory". I can't understand the first sentence there. It looks like this might have been broken in some editing process. Can anyone fix this paragraph and maybe expand on it?
-
- CommentRowNumber2.
- CommentAuthorTim_Porter
- CommentTimeFeb 23rd 2010
Thanks, Urs. That should probably go in a strong shape theory entry but goodness knows when I will have time to put one in. I have not read what you have said yet, but it seems to confirm what I thought was the case.
I have had a go at shape theory. It will need a bit more work to ease the new material in more seemlessly into the old.the problem was also at dense subcategory as the second definition did not quite make the link to other ideas and terminology that are used (and perhaps you are more to). This will require me to do a bit more work defining proadjoint and proreflective subcategory, and probably writing something about the links with profunctors/distributors as that is nearer the central methodology of the n-lab entries.
-
- CommentRowNumber3.
- CommentAuthorUrs
- CommentTimeFeb 23rd 2010
Thanks, Tim. I just saw what you did. I have edited the entry a bit more now, in an attempt to make the structure clearer:
I created one subsection "Abstract shape theory" with its Idea- , Definition- and Examples- section
I restructured the paragraph that defined the shape-category a bit, such as to make clear which bit of it gives the definition of the shape category, and which recalls just (one of) the definition(s) of dense subcategory.
then I made the example of profinite groups that you gave one item in a list of examples, whose currently only other item is that of topological spaces. Please check if I said correctly how shape theory for topological spaces is a special case of abstract shape theory.
then in the expectation that shape theory for topological spaces will be the example with most of the material associated to it, I created a second section after "Abstract shape theory" on "Shape theory for topological spaces". Currently that does not contain much besides the oo-stack treatment that I typed yesterday, but I hope eventually we can fill in something here.
Have a look and see if you agree with what I did.
-
- CommentRowNumber4.
- CommentAuthorUrs
- CommentTimeFeb 23rd 2010
- (edited Feb 23rd 2010)
Concerning the different definitions at dense subcategory: might it be better if we explicitly say -- as is suggested there -- pro-reflective subcategory instead of "dense subcategory in the second sense of the term"? Then we don't have the awkward "it is a dense subcategory, but not in the sense that you probably think"-problem. And "pro-reflective subcategory" is nicely descriptive.
-
- CommentRowNumber5.
- CommentAuthorTim_Porter
- CommentTimeFeb 23rd 2010
That would be my preference. We should however include the term 'dense' on the entry on proreflective, as the term is used.
I have done additional edits and tried to structure the references (and to be a bit fairer to my own contributions to the area :-).
-
- CommentRowNumber6.
- CommentAuthorMike Shulman
- CommentTimeFeb 23rd 2010
I would be in favor of making dense subcategory entirely about the other notion, with just a brief comment (and link) that the word is also sometimes used in shape theory to refer to a pro-reflective subcategory.
-
- CommentRowNumber7.
- CommentAuthorzskoda
- CommentTimeFeb 23rd 2010
Dense subcategory is not sometimes used in that meaning in shape theory but 95% of the time.
-
- CommentRowNumber8.
- CommentAuthorTim_Porter
- CommentTimeFeb 23rd 2010
Already in Categories for the Working Mathematician in 1971, one finds the first use of 'dense subcategory'. Mac Lane is not clear on the origin of the term. Sibe Mardesic uses the term (in the second sense) in some notes in 1978, (referred to in a paper by Luciano Stramaccia (archive.numdam.org/article/RSMUP_1982__67__191_0.pdf) where it proved that the shape theorists' use of the term was equivalent to pro-reflectivity).
I recall that in discussions with Bourn and Cordier in visits to Amiens in about 1980, we wasted a lot of time trying to work out what the condition was because it was not density as we knew it. Afterwards the other two realised what the point was (I took longer and needed it explained to me.) Shape theorists 'density' is very nearly the same as categorists 'codensity'. In the categorical form of 'dense', things can be nicely approximated by colimits of objects from the subcategory, whilst in the other they look like limits of such objects. The point becomes more important when you note, as did Bourn and Cordier, in 1980, that for a functor K, the shape theory of K is the Kleisli category of the codensity monad of K if this exists (and it will if we work with distributors/profunctors).
So the two notions are confusingly similar because they seem nearly dual to each other. This is unfortunate, but is a fact.
(Pro-adjoints predate all this as they are, I think, already in Grothendieck's descent seminars, where pro-categories are explored for the first time.)
The vast majority of uses of the term 'dense category' is in the first sense, as Mac Lane's book was the main source of reference for many years, and usually the terminology he used prevailed. (Look back at Mitchell's book on category theory and you have to unravel what his definitions correspond to in Mac Lane as they are sometimes the duals.) Many shape theorists use the second sense or both (there are papers that use 'pro-reflective (i.e. dense)' and so our use should also recognise that fact, however the present 'first sense' / 'second sense' method seems a mess and is likely to lead to more confusion, and I think it should be avoided if at all possible.
What is suggested is, I think, that
(i) in the shape theory pages the use of 'dense' be replaced by 'pro-reflective';
(ii) in the entry on dense subcategory, the discussion of the second use is replaced by a disambiguation phrase to the effect that: 'For the use of 'dense subcategory' in many papers on shape theory, please see 'pro-reflective subcategory, with of course a link';
(iii) new entries on 'pro-adjoints' and 'pro-reflective subcategory' be created (we need them anyway) and the second of these should briefly discuss the term 'dense' as used as a synonym for 'pro-reflective' by many shape theorists. This discussion should mention the motivation of that use, its history, etc.
-
- CommentRowNumber9.
- CommentAuthorzskoda
- CommentTimeFeb 23rd 2010
It is a good proposal.
-
- CommentRowNumber10.
- CommentAuthorTim_Porter
- CommentTimeFeb 24th 2010
@ Urs: I edited your entry on proreflective subcats of Top. That was not quite what is the case as the constructions either use the nerve (giving a Cech homotopy approach, say by myself or more generally by Morita using some neat methods) or use Sibe Mardesic and Jack Segal's approach via ANR-systems and resolutions, but in either case the important fact is that pro-HoCW is proreflective in pro-HoTop. That is what make it shape theory not strong shape theory. In strong shape everything has to be made homotopy coherent and that is what Batanin did in a categorical way and Mardesic and Lisica in an explicit topological construction (see his Strong homology book). My approach had been to use Vogt's theorem to realise Ho(pro-SSet) as a category of homotopy coherent diagrams (sort of).
-
- CommentRowNumber11.
- CommentAuthorUrs
- CommentTimeFeb 24th 2010
oh, I see, apply Ho first. Okay, thanks.
-
- CommentRowNumber12.
- CommentAuthorTim_Porter
- CommentTimeFeb 24th 2010
There is a notational problem I believe. I used HoTop to mean spaces mod homotopy equivalences, whilst Ho(Top) is mod W.es is it not. How should this be fixed?
-
- CommentRowNumber13.
- CommentAuthorUrs
- CommentTimeFeb 24th 2010
- (edited Feb 24th 2010)
I have expanded Ho(Top) now to account for this better. I write there
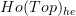 and
and 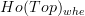 now.
now. -
- CommentRowNumber14.
- CommentAuthorTim_Porter
- CommentTimeFeb 24th 2010
Thanks. That looks good.
I will add an informal discussion of the Warsaw circle and how its weak homotopy type does not reflect its properties. My thought is that this might go well in a new entry rather than in a subentry to shape theory. What do others think?
-
- CommentRowNumber15.
- CommentAuthorUrs
- CommentTimeFeb 24th 2010
Sure, create a new entry and link to it.
-
- CommentRowNumber16.
- CommentAuthorTim_Porter
- CommentTimeFeb 25th 2010
- (edited Feb 25th 2010)
I have started on an entry on the Warsaw Circle (or one variant of it). Not got far yet. I think it may be a good idea to discuss the basic ideas of Borsuk shape, Cech homotopy, and ANR-systems in an example like this, and at an intuitive level, as I doubt we really need long detailed entries on all of them. For instance that this space has the same shape as a circle is nice and intuitively based, so would be good to give non-experts a taste for the idea. To put that in the main shape entry would clog it up. Another example to include in a separate entry may be the solenoids. Again picking out those features that help understand how shape works on them.
(I have a backlog of refereeing and reviewing which is giving me a guilty feeling so probably little will be done for a few days!)
-
- CommentRowNumber17.
- CommentAuthorTim_Porter
- CommentTimeFeb 27th 2010
One of my aims in doing this is to start on an investigation of how (strong) shape maps between simple spaces such as this one and the circle itself look when we view strong shape through the microscope provided by HTT and its relatives. Any thoughts on this would be appreciated. :-)
I have added a bit more to the entry just now.
-
- CommentRowNumber18.
- CommentAuthorUrs
- CommentTimeFeb 27th 2010
- (edited Feb 27th 2010)
when we view strong shape through the microscope provided by HTT and its relatives
Good point. We might get a nice example here. oo-stacks on the (Warsaw) circle are not that different from plain simplicial sheaves, because essentially only the double intersections of open subsets matter. So one should be able to write out things very explicitly here.
-
- CommentRowNumber19.
- CommentAuthorTim_Porter
- CommentTimeFeb 27th 2010
My hope is that not only should this work but it should be possible to d something similar for the dyadic solenoid! That is also well controlled in its construction, but is not stable, in the sense that the 'bonding maps' are not homotopy equivalences, so I have no way as yet of knowing what its HTT shape should be, hence my effort to describe in fairly simple terms the various ways of viewing these examples.
-
- CommentRowNumber20.
- CommentAuthorTim_Porter
- CommentTimeFeb 28th 2010
- (edited Feb 28th 2010)
I changed the title of ?ech methods to that. The accent was missing on the old version! Of course now the accent does not appear here!!!!!
-
- CommentRowNumber21.
- CommentAuthorTim_Porter
- CommentTimeFeb 28th 2010
I have started a new section on the Cech homotopy of the Warsaw circle.
-
- CommentRowNumber22.
- CommentAuthorMike Shulman
- CommentTimeMar 1st 2010
A while ago, perhaps in this thread, we discussed using what characters to use in page names, and we sort of decided on being fairly restrictive, although I think we didn't settle on a specific character set restriction. But you'll notice that "
 -category" redirects to "infinity-category" and not the other way 'round, for instance, so that the actual page title contains no unicode. I think that the original naming of Cech methods without the accent was in line with this convention. If we still agree on that convention, then we should probably record it in the FAQ, or we could of course have the discussion again (yay!).
-category" redirects to "infinity-category" and not the other way 'round, for instance, so that the actual page title contains no unicode. I think that the original naming of Cech methods without the accent was in line with this convention. If we still agree on that convention, then we should probably record it in the FAQ, or we could of course have the discussion again (yay!). -
- CommentRowNumber23.
- CommentAuthorTim_Porter
- CommentTimeMar 1st 2010
I seemed to recall something like that. The title works well. It was just the TOC.
-
- CommentRowNumber24.
- CommentAuthorMike Shulman
- CommentTimeMar 1st 2010
Not sure what you mean -- are you okay with changing the title of Cech methods back to without the accent?
-
- CommentRowNumber25.
- CommentAuthorTim_Porter
- CommentTimeMar 1st 2010
Yes whatever works best globally. I don't really mind, either is clear. My point was that there is apparently no great problem with the use of the accented C in the title. The systems seems to cope with that. Where is objects is in producing the table of contents if it is used in a section heading. There it crashes, which is an 'interesting reaction', which no doubt has some explanation involving different font systems being used by different parts of the software. The important thing is that it works!!!!
-
- CommentRowNumber26.
- CommentAuthorTim_Porter
- CommentTimeMar 1st 2010
I have added in several references to the shape theory of -algebras. This is something that may be worth pursuing. There are nice notes : Noncommutative topology - homotopy functors and E-theory that I have linked to there. They are quite old but are very nicely written. There is a discussion of model category structures on categories of -algebras if I remember rightly. Certainly they are worth glancing at.
-
- CommentRowNumber27.
- CommentAuthorzskoda
- CommentTimeMar 1st 2010
It is better with the accent
-
- CommentRowNumber28.
- CommentAuthorTim_Porter
- CommentTimeMar 1st 2010
I agree, but there are potential software problems because of the accent. For instance it does not work here. My policy will be to use the accent in as many places as do not cause problems. (I had one place with accented C in a section title and the program blocked me and I lost the text that I had taken 10 minutes to type!!! It was the table of contents part of the system that reacted badly.)
-
- CommentRowNumber29.
- CommentAuthorzskoda
- CommentTimeMar 1st 2010
- (edited Mar 1st 2010)
There is one frequent problem in nlab which I never understood: when I create a new entry, sometime I create an entry, having say 3 paragraphs and the very first is not seen on the output. Then I change something like add an empty line on the top or something until it works. Sometimes I can not start the page with certain sequence and until I change the order of the titles, links, sentences and so on I can not make it work. It happens once per say 15 new created entries.
Tim: It seems the site or something with E-theory lectures does not work either at the moment. As far as shape theory for C-star algebras, I have great appreciation of the work and expertise of Marius Dadarlat from Purdue University who worked on this pretty seriously (not fully and obviously reflected in his publication list).
-
- CommentRowNumber30.
- CommentAuthorTim_Porter
- CommentTimeMar 2nd 2010
Dadarlat's papers are of importance, yes. I will add the references.
That is strange about the Anderson-Grodal paper as I had checked the link when I edited the entry. (It is a nice introductory discussion.)
There is more that is needed on this E-theory stuff as I think it is the visible part of an 'iceberg' that is waiting to be revealed and which may be very important. I spent 7 year at UCC in Cork in Ireland back in the 1970s. The department at UCC was well known for Functional Analysis, and explored the links between shape theory and operator algebras (Brown-Douglas-Filmore) that were being made at that time.
-
- CommentRowNumber31.
- CommentAuthorTim_Porter
- CommentTimeMar 2nd 2010
Try that link from here.
http://www.math.ku.dk/~jg/papers/etheory.html
It worked for me, just 30 seconds ago!
-
- CommentRowNumber32.
- CommentAuthorzskoda
- CommentTimeMar 2nd 2010
Yes, today it works, yesterday it did not at all. Maybe the server...
-
- CommentRowNumber33.
- CommentAuthorTim_Porter
- CommentTimeMar 4th 2010
I have created a new entry on Borsuk shape theory. Please note the beautiful Chapman complement theorem stated there. It is one of the best duality results I know, there was a mention of it on the Café discussion either on Cech homotopy or on duality. I forget which.
-
- CommentRowNumber34.
- CommentAuthorTim_Porter
- CommentTimeNov 28th 2010
I just found that an old but useful paper by Dave Edwards is available online. As the Lab is out of action I will put the link here.
-
- CommentRowNumber35.
- CommentAuthorzskoda
- CommentTimeJan 20th 2011
- (edited Jan 21st 2011)
I have changed the organization and some text in shape theory. I put an idea section separate from motivation. Motivation is about examples of bad spaces and inadequacy of homotopy groups. The idea is more about where the maps go and how to correct. I dislike the standard phrase that the homotopy theory is bad for locally bad spaces. I mean it is ridiculous as the shape is the invariant of strong homotopy type. I mean the thing which is bad is that the homotopy theory is much developed only for calculating the weak homotopy type. So in a way the thing is how to quotient out the strong homotopy type to something manageable. If one quotient it to weak homotopy type, then no difference for good spaces and disaster for bad spaces. If one quotients it to (strong) shape then the things are better for such.
Another thing is that Friedrich Bauer had a paper in 1985 (in a Georgian journal)
- F. V. Bauer, Strong shape theory. (Russian. English summary) Trudy Tbiliss. Mat. Inst. Razmadze Akad. Nauk Gruzin. SSR 77 (1985) MR87m:55009
where the strong shape is defined in terms of strict -categories (with the invertibility of -cells for ). Note that this was 1985. Günther had later made a refined variant of Bauer’s approach in his thesis under Bauer, around 1991, where he translated the theory into more natural language of simplicial sets (emphasising on weak Kan conditions and hence on what we now call quasicategories). A more complete account is
- Bernd Günther, The use of semisimplicial complexes in strong shape theory. Glas. Mat. Ser. III 27(47) (1992), no. 1, 101–144, MR94g:55014, googlebooks
Lurie says in HTT that his approach is from Toen-Vezzosi, where the latter in 2002 looked into the subject from the point of view of Segal categories. But Simpson had looked into shape from a similar point of view in passing for algebraic geometry applications in late 1990s. In any case, Lurie quotes the Guenther’s paper in his book, but does not say about the relation of approaches.
-
- CommentRowNumber36.
- CommentAuthorTim_Porter
- CommentTimeJan 20th 2011
Thanks Zoran.
Friedrich Wilhelm Bauer had several papers along those lines at the time and Guenther’s thesis was good. (I may still have a copy although much of my old archive has been thrown out due to lack of space.) He was mayor of Frankfort as well as a professor of mathematics, so I always found it amazing that he could find the time to work on shape theory. I did not like his approach that much as it was at the same time categorical in flavour but did not use that much of the available category theory. If I have copies I will try to summarise the method. (I had copies in English of longer papers,)
-
- CommentRowNumber37.
- CommentAuthorzskoda
- CommentTimeJan 21st 2011
- (edited Jan 21st 2011)
I corrected slightly the entry 35 and added the link to the Günther’s paper which translated Bauer’s ideas into more flexible simplicial language of weak Kan fibrations and related notions. Bauer was a mayor of Frankfurt or Günther ? Added a stub for Friedrich Wilhelm Bauer.
-
- CommentRowNumber38.
- CommentAuthorTim_Porter
- CommentTimeJan 21st 2011
He does not appear in the list of mayors on Wikipedia (which says it is incomplete but…). I have added several more of his students.
-
- CommentRowNumber39.
- CommentAuthorTim_Porter
- CommentTimeJun 12th 2012
- (edited Jun 12th 2012)
I have added some links to connections between shape theory and dynamical systems.
-
- CommentRowNumber40.
- CommentAuthorzskoda
- CommentTimeJun 18th 2016
Sad news today. One of the main researchers of shape theory, member of Croatian Academy of Sciences (HAZU), Prof. Sibe Mardešić passed away last night, several days short of 89. birthday (june 20, what will be on Sunday).
-
- CommentRowNumber41.
- CommentAuthorTim_Porter
- CommentTimeJun 18th 2016
- (edited Jun 18th 2016)
Please, Zoran, can you include me in any condolences that you send to Zagreb university, to the Academy of sciences, etc. I met Sibe several times and was always impressed by his courtesy, kindness and friendship.
His intuition about the importance of the shape theoretic approach has be shown to be justified in full generality.
-
- CommentRowNumber42.
- CommentAuthorzskoda
- CommentTimeApr 3rd 2017
I added the early reference by Holsztyński at shape theory.
-
- CommentRowNumber43.
- CommentAuthorDavidRoberts
- CommentTimeJul 24th 2019
-
- CommentRowNumber44.
- CommentAuthorUrs
- CommentTimeJun 15th 2020
I have added mathscinet pointer for
- K. Borsuk, Theory of Shape, Monografie Matematyczne Tom 59, Warszawa 1975 (mathscinet:0418088)
But is there an y more explicit electronic pointer to this article? Best would be a scanned pdf, but mayebe we have at least an ISBN or DOI for a publication, maybe in a “collected works”-compendium, or similar?
-
- CommentRowNumber45.
- CommentAuthorDavid_Corfield
- CommentTimeJun 15th 2020
There’s the identically named
- K. Borsuk, Theory of shape (Lecture Notes Series, 28. MatematiskInstitut, Aarhus Universitet, Aarhus, 1971. Reprinted, 1973)
Don’t know if that differs.
-
- CommentRowNumber46.
- CommentAuthorDavid_Corfield
- CommentTimeJun 15th 2020
Added
- Karol Borsuk, Jerzy Dydak, What is the theory of shape?, Bulletin of the Australian Mathematical Society, 1980, 22(2), 161-198, (doi:10.1017/S000497270000647X)
-
- CommentRowNumber47.
- CommentAuthorUrs
- CommentTimeJun 15th 2020
Thanks!
Do you know a publication, page, and verse that one could usefully point to for the statement that the shape of nice enough topological spaces coincides with their usual homotopy type?
I’d like something explicit to point to for justifying writing “shape” for ordinary homotopy type.
-
- CommentRowNumber48.
- CommentAuthorDavid_Corfield
- CommentTimeJun 15th 2020
There’s some history at the start of Theory of Compact Hausdorff Shape. Don’t know if that helps? But Tim Porter was actively involved in this, so could surely say.
I hadn’t realised that the ’Segal’s on this page refer to Jack, except ’Segal categories’ mentioned in one reference, and that’s Graeme.
-
- CommentRowNumber49.
- CommentAuthorTim_Porter
- CommentTimeJun 15th 2020
- (edited Jun 15th 2020)
The reference K. Borsuk, Theory of Shape, Monografie Matematyczne Tom 59, Warszawa 1975 (mathscinet:0418088) is to a quite sizeable book and is probably not available as an e-book, but I have not checked.
The story of the result that says ‘nice enough’ topological spaces have shape which coincides with their homotopy type is quite complicated. Possibly the best results are in D.A. Edwards and R. Geoghegan, Stability theorems in shape and prohomotopy, Trans. AMer Math. Soc. 222 (1976) 389 - 403.
The term Stability refers to a pro-object being equivalent to a constant one. There is also a paper by Ross Geoeghegan in General Topology and its Applications 8 (1978) 265-281.
There is also a paper by me: Stability results for topological spaces. Math. Z. 140 (1974), 1-21 which handles other forms of stability e.g. rational stability. There are also some results that relate local topological properties of a space to stability. (I have a large almost comprehensive archive of shape theory and strong shape theory papers (up to perhaps the mid to late 1980s, but they are in boxes upstairs!!!!! )
I thought that the results on that problem were not that important these days as they date from the 1970s, and somehow the results were ’sealed’ as they did not lead to further things except that they did give rise to Strong Shape. Otherwise I would have added things to the n-lab on this (and can still do so if it is useful, although the methods used are somewhat ’old hat’ now.))
-
- CommentRowNumber50.
- CommentAuthorTim_Porter
- CommentTimeJun 15th 2020
- (edited Jun 15th 2020)
@48. Yes I have now! If more is thought useful, as I said in the reply above, I can supply more and would be happy to do so.
-
- CommentRowNumber51.
- CommentAuthorUrs
- CommentTimeJun 15th 2020
The reference K. Borsuk, Theory of Shape, Monografie Matematyczne Tom 59, Warszawa 1975 (mathscinet:0418088) is to a quite sizeable book and is probably not available as an e-book, but I have not checked.
Thanks. But does the book have a a title and an ISBN? Or anything witnessing its existence?
Possibly the best results are in D. A. Edwards and R. Geoghegan, Stability theorems in shape and prohomotopy, Trans. AMer Math. Soc. 222 (1976) 389 - 403.
Thanks! I’ll try to get hold of this and dig through it.
Or might you have a fully explicit pointer for me, to page number and/or theorem number?
I don’t necessarily need the the original article(s), in fact would prefer a clean-ed up review that one can send readers to without causing them harm.
-
- CommentRowNumber52.
- CommentAuthorTim_Porter
- CommentTimeJun 15th 2020
I have dug out my copy of Brosuk’s book and there is not even a Library of Congress number. A Google search found ISBN numbers on Amazon: ISBN-13: 978-0800223434 ISBN-10: 0800223438 The publisher was The Polish Academy of Sciences (Polish Scientific Publishers.)
I don’t understand your question about its title as that is ’Theory of Shape’.
There is not that much in Borsuk’s book about the stability problem, merely a summary towards the end.
There is a reasonably accessible account of the Stability Problem in section 5.5 of D.A. Edwards and H. M. Hastings, (1976), Čech and Steenrod homotopy theories with applications to geometric topology, Lecture Notes in Maths. 542, Springer-Verlag. That may be a good source to direct readers to.
-
- CommentRowNumber53.
- CommentAuthorUrs
- CommentTimeJun 15th 2020
I thought that the results on that problem were not that important these days
I admit that here I am not interested in the results of shape theory… but just in stealing the word “shape” – as a synonym for “homotopy type of a topological space” in the case that the topological space is nice enough.
We have been doing this already here for a long time. Now I’d just like to add a proper hat-tip citation in order to compensate for this linguistic appropriation.
-
- CommentRowNumber54.
- CommentAuthorTim_Porter
- CommentTimeJun 15th 2020
I had noticed the use that Lurie had made of the term. It seems fine to me to use it as you have been doing as long as the spaces concerned have the homotopy type of CW-compex or an (F)ANR then Shape and homotopy type coincide. On the other hand I think Lurie’s use of rthe term
singular shape' is not a good one as it seems to meansame shape as its singular complex’ for him, whilst the term ‘singular shape’ might be read as saying that the shape had singularities, such as a solenoid. (Note a Warsaw circle is stable as it has the shape of a circle. Stability is about the behaviour of approximations to the shape by nerves of open covers. In a stable shape, the idea is that the homotopy type of the nerves does not change with refinement and the linking maps are homotopy equivalences.)As I said I did not develop the shape theory pages in the Lab because it did not seem necessary for the development of the Lab to have lots of pages that were of historical interest only.
-
- CommentRowNumber55.
- CommentAuthorMarc
- CommentTimeJun 15th 2020
-
- CommentRowNumber56.
- CommentAuthorUrs
- CommentTimeJun 15th 2020
It seems fine to me to use it as you have been doing as long as the spaces concerned have the homotopy type of CW-compex
Yes, I know, but I am looking for a concrete citation of this fact.
-
- CommentRowNumber57.
- CommentAuthorTim_Porter
- CommentTimeJun 15th 2020
- (edited Jun 15th 2020)
I do not exactly know what you are seeking.
The problem is that ’homotopy type’ has the same multiplicity of related meanings as does ‘shape’. Is the homotopy type of a space, , the collection of spaces for which there is a homotopy equivalence from that space to ? Is the shape of a topological space, , the collection …. shape equivalence to . Even if is a simplicial complex these are not the same, as it depends in what category you are working. If you are considering that ’space’ means ’space having the homotopy type of a simplicial complex’ then the collections are the same, but if you need to consider a larger category of ’spaces’ they may not be. As I said the Warsaw circle has the same classical shape as a circle but not the same homotopy type.
I said about the case in which ’space’ means ’having the homotopy type of a simplicial complex’. That is a consequence of the fact that open covers of such a space can be refined to be finer than any triangulation and vice versa, and that is very classical, 1930s topology. (The Cech nerve stabilises to the space in any of the forms of shape theory.)
There is thus no result that says that for such and such a space the two concepts always coincide as it depends on the ambient category of ’spaces’. The results I was pointing you two relate to the standard forms of classical shape theory in which the spaces are compact metric, or compact Hausdorff. There they give characterisations of those spaces that have the same shape as a CW-complex.
The extensions of ’shape’ to more general geometric objects or to toposes etc, still will have the same problem. In a given context that will be hopefully clear.
-
- CommentRowNumber58.
- CommentAuthorUrs
- CommentTimeJun 15th 2020
Tim, you claimed in #54:
as the spaces concerned have the homotopy type of CW-compex or an (F)ANR then Shape and homotopy type coincide
Please give a citation for this statement.
-
- CommentRowNumber59.
- CommentAuthorTim_Porter
- CommentTimeJun 15th 2020
I think I would prefer to retract that statement as it is. (in fact I was miss remembering the theory when I mentioned FANRs!) What is true almost axiomatically is that two CW-complexes have the same shap if and only if they have the same homotopy type. (You will want a citation for that! It is a consequence of Theorem 3.2.1 of Dydak and Segal, Shape theory An introduction, Springer Lecture Notes 688, but the result is in many other sources, but not always that visible.)
-
- CommentRowNumber60.
- CommentAuthorUrs
- CommentTimeJun 15th 2020
-
- CommentRowNumber61.
- CommentAuthorUrs
- CommentTimeAug 2nd 2020
added pointer to
- Jintao Wang, Theorem 4.6 of: Theory of Compact Hausdorff Shape (arXiv:1708.07346)
for an explicit statement of shape coinciding with homotopy type (here for compact Hausdorff spaces)
-
- CommentRowNumber62.
- CommentAuthorUrs
- CommentTimeOct 4th 2021
- (edited Oct 4th 2021)
Where is an actual proof spelled out that for a paracompact space the shape of the -topos “is” the strong shape of in the classical sense?
The section here titled “Strong shape via -topos theory” contains nothing but a kind of rough review of To¨n \& Vezzosi’s and Lurie’s definitons, but no pointer to any classical shape theory that this would connect to. The material the section does contain is, at least meanwhile, much better done at shape of an (infinity,1)-topos, and I suggest we delete this section here and replace it by a pointer to shape of an (infinity,1)-topos together with some actual discussion relating to classical shape.
In fact, HTT does not seem to explicitly claim to reproduce definitions of authors in classical strong shape theory, it just has a Remark (7.1.6.7) which speaks about “conforming terminology”. Similarly, Toën-Vezzosi make no comment on how their definition relates to classical definitions (they don’t even mention classical shape theory nor cite anyone, they just let the term fall from the sky).
I am not doubting it, but can we point to page and verse in a classical strong shape text where one finds a definition that could usefully be compared to the -topos theoretic formulation?
-
- CommentRowNumber63.
- CommentAuthorMarc Hoyois
- CommentTimeOct 5th 2021
Remark 2.13 in my paper Higher Galois theory explains why the topos shape agrees with the shape of compact Hausdorff spaces as defined by Mardešić and Segal (http://matwbn.icm.edu.pl/ksiazki/fm/fm72/fm7214.pdf). I don’t know the classical definitions beyond this case.
-
- CommentRowNumber64.
- CommentAuthorUrs
- CommentTimeOct 5th 2021
-
- CommentRowNumber65.
- CommentAuthorUrs
- CommentTimeOct 5th 2021
(But the entry shape theory remains a mess. Despite making several apparent attempts to say something, it doesn’t.)
1 to 65 of 65