nForum
Not signed in
Want to take part in these discussions? Sign in if you have an account, or apply for one below
Site Tag Cloud
Vanilla 1.1.10 is a product of Lussumo. More Information: Documentation, Community Support.
If you want to take part in these discussions either sign in now (if you have an account), apply for one now (if you don't).
-
- CommentRowNumber1.
- CommentAuthorTim_Porter
- CommentTimeFeb 12th 2010
I have started adding stuff to homotopy coherent diagram I have added new references into Rainer Vogt and have created Jean-Marc Cordier.
-
- CommentRowNumber2.
- CommentAuthorUrs
- CommentTimeFeb 12th 2010
Thanks! I added a toc.
-
- CommentRowNumber3.
- CommentAuthorTodd_Trimble
- CommentTimeFeb 12th 2010
I don't want to write over what Tim is doing, but I personally find it congenial to define a homotopy coherent diagram
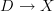 (
( an ordinary category,
an ordinary category,  a simplicial category) to be a simplicial functor
a simplicial category) to be a simplicial functor 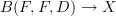 where
where 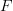 is the free category on a graph monad, and
is the free category on a graph monad, and 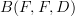 indicates a two-sided bar construction. Any chance this POV can be incorporated into the article?
indicates a two-sided bar construction. Any chance this POV can be incorporated into the article? -
- CommentRowNumber4.
- CommentAuthorTim_Porter
- CommentTimeFeb 12th 2010
I am having strange losses of comments!! I replied to this very fully earlier and the comments appeared but is no longer here.
Yes Todd, The entry so far is just the intro. It is more or less exactly the POV which will be given as the definition in general. I will give Vogt's definition as a half way stage, but then the simplicial resolution / bar construction will be given.
-
- CommentRowNumber5.
- CommentAuthorTodd_Trimble
- CommentTimeFeb 13th 2010
Okay, great -- thanks, Tim.
-
- CommentRowNumber6.
- CommentAuthorUrs
- CommentTimeFeb 13th 2010
- (edited Feb 13th 2010)
I think generally we allow ourselves to add further perspectives to an entry without that being either intended or regarded as "writing over" previous material. Thre is always room for a new subsection giving one more perspective.
-
- CommentRowNumber7.
- CommentAuthorTim_Porter
- CommentTimeFeb 13th 2010
I agree. My plan is to introduce Vogt's homotopy description. then link with bar resolution / simplicial resultion, and the h.c. nerve finally letting loose a whole lot of links to quasicats etc.
-
- CommentRowNumber8.
- CommentAuthorTodd_Trimble
- CommentTimeFeb 13th 2010
@Urs: yes, I agree generally, but insofar as Tim is a guru on this subject and has ideas on how this should be expounded, I thought I should wait and ask first. Sounds like a great plan, Tim.
-
- CommentRowNumber9.
- CommentAuthorUrs
- CommentTimeFeb 13th 2010
- (edited Feb 13th 2010)
I have a suggestion, too:
to me, the most transparent conceptual way to think of a homotopy coherent diagram
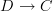 is that it is a model for a functor of (oo,1)-categories.
is that it is a model for a functor of (oo,1)-categories.If we think of these as modeled by simplicially enriched categories, then what Todd indicates above should produce the required cofibrant replacement of
 .
.Would you agree with that, Tim?
-
- CommentRowNumber10.
- CommentAuthorTim_Porter
- CommentTimeFeb 13th 2010
I have a pedagogic reaction to that, so I think that that is the end point of an exposition rather than the initial one. I have given lots of talks on h.c. diagrams and have found that people (i.e. mathematicians, even algebraic topologists, who are not au fait with the ideas) are even amazed how they arise in that simple situation where you have take a commuting triangle of spaces, and maps, and replace each space by homotopy equivalent one. i.e. bare hands work! There are lots of such people and if they face a high cliff of ideas to face up to the concept then they will shut it out. I therefore suggest that we put the ideas that you suggest towards the end of a discussion so that the bare hands stuff helps 'people' to understand functors between (oo,1)-categories.
I faced a lot of opposition from the mathematical 'nomenclatura' when I tried to get grants to follow the quasicategory line in the 1980s. (As I repeat, Jean-Marc and I sketched out the basics on a walk on Anglesey in about 1984 or there abouts. We applied but did not get funding and the extreme reaction against the ideas from some referees was very hurtful. We did try to do too much using simplicial enriched categories rather than quasicategories as we thought the latter would not be accepted at that time. Now things may be different and the homotopy theorists are much more positive about this viewpoint.
So as yet I think of h.c. as a way to explain the (oo,1)-terminology and to give a feel of why that is useful and very important.I may be wrong but I remember a reaction of an influential professor when I explained crossed complexes in terms of simplicial groups and as he understood where those lay in relation to stuff he knew, he was happy. I think it expedient to start where those 'people' are and make them feel they understand where we are rather than to go too quickly to the elegant approach.
That is the pedagogic reply, now the maths. I believe that what you say is completely right. I feel that one thing the h.c. nerve does is link up the bar construction more explicitly with other notions of h.c. There is a mysterious reference by Dwyer and Kan to the simplicial resolution as giving infinitely homotopy commutative diagrams, as if that was obvious, and to me there was a lot of detail to check and to check explicitly.
-
- CommentRowNumber11.
- CommentAuthorTim_Porter
- CommentTimeFeb 13th 2010
@Urs: Your comment clarified something for me . Thanks. It has only taken me 25 years to realise something. (There is hope yet!) I can build in more of your viewpoint and what I might call Cordier-Trimble earlier in the entry I think and it will make it more coherent (pun intended) with the rest.
-
- CommentRowNumber12.
- CommentAuthorUrs
- CommentTimeFeb 13th 2010
Thanks for the reply, Tim. I am perfectly happy with having the entry go the route that you envision.
I wish I could somehow alleviate the feelings you have about your early important work getting such an ignorant reaction. But since I can't turn back the time, this is difficult. Maybe the best thing one can do now is to amplif how very right you and Cordier have been then and over all these years. And for this it is good to point out how what you did is related, closely related, or identical to what is done now.
-
- CommentRowNumber13.
- CommentAuthorTodd_Trimble
- CommentTimeFeb 13th 2010
- (edited Feb 13th 2010)
One further thought about this: the simplicial resolution
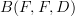 is not just any cofibrant replacement, it is a universal cofibrant replacement. (I've been reading a bit of Richard Garner's A homotopy-theoretic universal property of Leinster's operad for weak
is not just any cofibrant replacement, it is a universal cofibrant replacement. (I've been reading a bit of Richard Garner's A homotopy-theoretic universal property of Leinster's operad for weak  -categories and feel he makes some very pertinent remarks on cofibrant replacements and on arguing for choosing them canonically; see the discussion on page 2.)
-categories and feel he makes some very pertinent remarks on cofibrant replacements and on arguing for choosing them canonically; see the discussion on page 2.) -
- CommentRowNumber14.
- CommentAuthorTim_Porter
- CommentTimeFeb 13th 2010
@Urs: Don't worry about my moans!! One of my non-moaning reasons for emphasising that stuff from back in that period is that I think that there is risk that useful results and quite simple 'constructive' proofs get forgotten being replaced by the transfinite induction / small object argument type results and this seems a shame. There are very useful results which have more chance of being extended to new contexts because of the geometric nature. In as much as I understand Zoran's doubts about the overall picture I think that this may what he is thinking. This does not detract from the good things proved using model categories etc. but they do not apply in all interesting cases sometimes for elementary reasons.
-
- CommentRowNumber15.
- CommentAuthorTim_Porter
- CommentTimeFeb 13th 2010
@Todd: I have brought in the bar construction more explicitly in the description of the homotopy coherent nerve, so as to bring in that version of the construction. (I had always thought of it as being the comonadic simplicial resolution and not the bar construction.) Can you check that you are happy with that change. (Perhaps someone should create a stub for simplicial resolution, and something about the universality properties in general and in this particular case.)
-
- CommentRowNumber16.
- CommentAuthorTim_Porter
- CommentTimeFeb 13th 2010
I have fixed some typos (e.g. sSet instead of SSet, and $\Delta^n$ in certain places instead of [n]) please check for new ones!!!!
-
- CommentRowNumber17.
- CommentAuthorTim_Porter
- CommentTimeFeb 13th 2010
I have added Vogt's explicit definition of h.c. diagram.
-
- CommentRowNumber18.
- CommentAuthorTodd_Trimble
- CommentTimeFeb 14th 2010
Tim, I think I'm happy with the change -- thank you for incorporating that. I really like how this is shaping up.
As you know, I wrote up a long blog article on the comonadic simplicial resolution and gave a slightly idiosyncratic meaning to the universality, but other formulations for the universality are of course possible and are proven by adapting the acyclic models theorem. I may import and rework that article for the nLab, but in a few hours I'll be on vacation and I'm not sure what time I'll have for this in the next seven days.
-
- CommentRowNumber19.
- CommentAuthorTim_Porter
- CommentTimeFeb 14th 2010
Enjoy your vacation. Are you going 'to the sun'? I was going to say ' or to the snow' but you probably have too much of that anyway.
I think the idea of using an edited version of the blog entry if excellent. I feel that some linking up to older work by Barr-Beck and Duskin, might be a good thing as for instance Jack's work in his Memoir is a very valuable resource. Also Paul Glenn's thesis, and various things by Myles.
-
- CommentRowNumber20.
- CommentAuthorTodd_Trimble
- CommentTimeFeb 14th 2010
Yes, Florida! It's supposed to be "cold" down there (which is to say in the 60s Fahrenheit, perhaps 15-20 celsius or so).
Thanks for the advice! Pointers to the literature would be very welcome, since in this case I hardly know it at all.
-
- CommentRowNumber21.
- CommentAuthorDavidRoberts
- CommentTimeFeb 15th 2010
@Urs,
I like your description very much - it links up with the idea of weak n-functors being anafunctors with strict n-functors for legs, the source-leg being some free cofibrant replacement. -
- CommentRowNumber22.
- CommentAuthorTim_Porter
- CommentTimeFeb 15th 2010
Your right. At the time when we we developing this initially, we thought of the span/distributor idea as a means of expressing ideas about this but it did not seem as useful as it now does. I will try to remember to put that viewpoint in (and if I forget I am sure someone else will not!).
-
- CommentRowNumber23.
- CommentAuthorUrs
- CommentTimeFeb 15th 2010
@Todd,
wish you a nice vacation! It would be very nice if at some point we could have more of your bar resolution article and its relation to the stuff Garner talks about on the nLab. That would be very good.
-
- CommentRowNumber24.
- CommentAuthorUrs
- CommentTimeFeb 15th 2010
- (edited Feb 15th 2010)
@David
thanks. I hope it won't spoil your and Tim's enthusiasm if I point out that this perspective with some details is discussed in section 1.2.6 Homotopy Commutativity vs Homotopy Coherence in HTT.
By the way, Tim: at least in the latest version of HTT Cordier is cited right at the beginning of the section on the homotopy coherent nerve.
-
- CommentRowNumber25.
- CommentAuthorTim_Porter
- CommentTimeFeb 15th 2010
I realise that. Jacob was very correct in his reaction (even if he never replied to my e-mail telling him the history!)
I did get irritated when I got a paper to referee (from someone else) who reinvented the construction and had a footnote that mentioned that Lurie had the same idea.
I see no difficulty with HTT section 1.2.6. and will look at it again to see if I can link in our n-Lab entry to things there. My objections are when the scholarship or lack of it in whatever source, fails to even attempt to attribute ideas or results reasonably accurately. (For an extreme case read Recoltes et Semailles.)
1 to 25 of 25