Not signed in (Sign In)

Not signed in

Want to take part in these discussions? Sign in if you have an account, or apply for one below

  • Sign in using OpenID

Site Tag Cloud

2-category 2-category-theory abelian-categories adjoint algebra algebraic algebraic-geometry algebraic-topology analysis analytic-geometry arithmetic arithmetic-geometry book bundles calculus categorical categories category category-theory chern-weil-theory cohesion cohesive-homotopy-type-theory cohomology colimits combinatorics complex complex-geometry computable-mathematics computer-science constructive cosmology deformation-theory descent diagrams differential differential-cohomology differential-equations differential-geometry digraphs duality elliptic-cohomology enriched fibration foundation foundations functional-analysis functor gauge-theory gebra geometric-quantization geometry graph graphs gravity grothendieck group group-theory harmonic-analysis higher higher-algebra higher-category-theory higher-differential-geometry higher-geometry higher-lie-theory higher-topos-theory homological homological-algebra homotopy homotopy-theory homotopy-type-theory index-theory integration integration-theory internal-categories k-theory lie-theory limits linear linear-algebra locale localization logic mathematics measure measure-theory modal modal-logic model model-category-theory monad monads monoidal monoidal-category-theory morphism motives motivic-cohomology nlab noncommutative noncommutative-geometry number-theory of operads operator operator-algebra order-theory pages pasting philosophy physics pro-object probability probability-theory quantization quantum quantum-field quantum-field-theory quantum-mechanics quantum-physics quantum-theory question representation representation-theory riemannian-geometry scheme schemes set set-theory sheaf simplicial space spin-geometry stable-homotopy-theory stack string string-theory superalgebra supergeometry svg symplectic-geometry synthetic-differential-geometry terminology theory topology topos topos-theory tqft type type-theory universal variational-calculus

Vanilla 1.1.10 is a product of Lussumo. More Information: Documentation, Community Support.

Welcome to nForum
If you want to take part in these discussions either sign in now (if you have an account), apply for one now (if you don't).

nLab > Latest Changes: Answer to the query over at structured (infinity,1)-topos?

Bottom of Page

1 to 73 of 73

  1.  
    • CommentRowNumber1.
    • CommentAuthorHarry Gindi
    • CommentTimeApr 22nd 2010
    • (edited Apr 22nd 2010)

    Mike left a query box over at structured (infinity,1)-topos about admissibility structures. I am pretty sure that the admissibility structure is not, as the statement in the article says, a grothendieck topology. Rather, it is a class of morphisms that is in some way compatible with the grothendieck topology. At least looking at Toën's notes (which it seems are essentially a version of HAG II restricted to ordinary categories and ordinary stacks (I'm not positive that this is fully accurate, but I'm reasonably confident in the statement)), a geometric structure is a class of morphisms that is compatible with the grothendieck topology satisfying a number of conditions (that seem to match the axioms for an admissibility structure given here!). Correct me if I'm wrong, but it appears that an admissibility structure is precisely the class of morphisms P in the definition of a geometric context (or maybe even the pair (τ,P)).

    Here's the link. Anyway, if this is true, it appears to answer Mike's question (once suitably generalized to (∞,1)-categories).

    If I'm mistaken, please let me know.

    I've put this in the (Latest Changes) category because at the moment, there is no nLab general category.

    • CommentRowNumber2.
    • CommentAuthorUrs
    • CommentTimeApr 22nd 2010

    Thanks for highlighting this again, I had forgotten about it. Let me find a free minute, and I try to look into it. Might not be before tomorrow evening, though.

    • CommentRowNumber3.
    • CommentAuthorUrs
    • CommentTimeApr 23rd 2010

    Okay, I have half an hour now and will look into this.

    One question, you mention:

    At least looking at Toën's notes

    Which ones? The Barcelona lecture notes? Could you give link, page and verse of what you have in mind?

    One remark:

    There are indications that the existing notion of admissibilty structure is a bit too rigid. In David Spivak's thesis-the-published-version, there is a remark on a remark on this on page 62.

    • CommentRowNumber4.
    • CommentAuthorUrs
    • CommentTimeApr 23rd 2010
    • (edited Apr 23rd 2010)

    I am pretty sure that the admissibility structure is not, as the statement in the article says, a grothendieck topology.

    The statement in the article (which is manifestly left incomplete, as indicated by the ellipsis, at least until I'll have it expanded in a few minutes) is direct reproduction of the remark 1.2.4 in Structured Spaces.

    • CommentRowNumber5.
    • CommentAuthorUrs
    • CommentTimeApr 23rd 2010

    Time runs.

    I put in a complete definition of admissibility structure in structured (infinity,1)-topos. But already have run now, to catch my train. More later.

    • CommentRowNumber6.
    • CommentAuthorMike Shulman
    • CommentTimeApr 23rd 2010

    Thanks. I figured out the answer to my question: left-exactness is already part of the definition, separately from the admissibility structure. So in the case of ordinary ringed spaces, there is no additional admissibility structure, unless you want to talk about locally ringed spaces.

    I think we still need to figure out exactly how this is related to geometric theories. The idea of admissibility structure currently seems kind of ad hoc to me, and unnecessary since a geometric theory can be presented using just a site, no need to single out any special morphisms.

    • CommentRowNumber7.
    • CommentAuthorHarry Gindi
    • CommentTimeApr 23rd 2010
    • (edited Apr 23rd 2010)

    If you read Toën's notes, I'm pretty sure that the notion of a geometric context in his sense cannot be presented by a site, per se. For example, the theory of differentiable manifolds with the grothendieck topology given by local diffeomorphisms and the geometric structure given by differentiable submersions is a perfectly useful geometric theory, but I don't see how to present it as a site.

    • CommentRowNumber8.
    • CommentAuthorUrs
    • CommentTimeApr 23rd 2010

    I am wondering about this: except for the 2-out-of-3 condition, the definition of admissibility structure (in the secod equivalent version that I put into the nLab entry) essentially just says: Grothendieck topology generated from a coverage.

    • CommentRowNumber9.
    • CommentAuthorMike Shulman
    • CommentTimeApr 24th 2010

    Apparently my most recent edit destroyed all the unicode (now reverted). Chrome is worse than I thought; from now on I guess I do all my nlab editing in Firefox. (The reason I use Chrome when I can is that Firefox has a tendency to make my computer freeze.)

    @Urs #8: Yes, and the condition to be a structure sheaf essentially just says: cover-preserving lex functor. There are some situations, though, in which one wants to remember not just the Grothendieck topology but a coverage or pretopology generating it, though; could something of that sort be going on here?

    • CommentRowNumber10.
    • CommentAuthorHarry Gindi
    • CommentTimeApr 24th 2010

    @Mike: I can't see how that could be true. In algebraic geometry, the geometry on the category of étale stacks on Aff is given by the class of smooth morphisms, not étale morphisms, and the smooth topology is strictly finer than the étale topology.

    • CommentRowNumber11.
    • CommentAuthorMike Shulman
    • CommentTimeApr 24th 2010

    Well, the definition on the page says that “all covering sieves are generated from admissible morphisms.” Shouldn’t that imply that a functor which preserves covering sieves of admissible morphisms also preserves all covering sieves?

    • CommentRowNumber12.
    • CommentAuthorHarry Gindi
    • CommentTimeApr 24th 2010

    I think that the trick here is that there are actually two grothendieck topologies that we're working with, where the admissible topology is generated by morphisms that are compatible with the descent topology.

    • CommentRowNumber13.
    • CommentAuthorMike Shulman
    • CommentTimeApr 24th 2010

    So are you saying the definition on the page isn’t correct as stated? Or am I reading it wrong?

    • CommentRowNumber14.
    • CommentAuthorHarry Gindi
    • CommentTimeApr 24th 2010
    • (edited Apr 25th 2010)

    I just looked it up in structured spaces, and the definition is incorrect as stated on the nLab page. An admissible structure consists of a subcategory and a grothendieck topology on that subcategory. This is a well-worthwhile distinction from what the nLab page says. In particular, this now actually makes sense in both the algebraic and differential contexts.

    We take (for algebraic geometry) the smooth subcategory to be the admissible subcategory (same objects but the morphisms are smooth morphisms) and equip it with the étale topology, where the covering sieves are generated by jointly strict epimorphic (every base change of the map induced on the coproduct is surjective) collections of étale morphisms 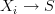X_i \to S. (The strict epimorphism condition guarantees that the topology is subcanonical).

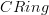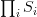    (Note that this corresponds in CRing to collections of étale algebras R\to S_i such that their product \prod_i S_i is a faithfully flat R-algebra).

    Using the correct definition, this now makes sense because every étale morphism is smooth.

    The differential context is similar in that every local diffeomorphism is a submersion, so we take our admissible subcategory of the category of "open sets in R^n" with morphisms being differentiable to be the subcategory generated by submersions and give the grothendieck topology as jointly surjective families of local diffeomorphisms.

    I believe that Toën's definition of a geometry (suitably generalized to (infinity,1)-categories) is strictly more general (although who knows whether or not this generality is worth the trouble) in that the class of geometric structure morphisms need not contain the maps generating the grothendieck topology. This is most likely the reason why his definition of a geometric context is so much longer, namely that the definition has removed the requirement that all morphisms in \tau are also in P.

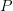    By the way, Mike, I'm not so sure that you were mistaken, but what I've said here should explain to Urs what was wrong with this statement:

    I am wondering about this: except for the 2-out-of-3 condition, the definition of admissibility structure (in the second equivalent version that I put into the nLab entry) essentially just says: Grothendieck topology generated from a coverage.

    The problem with this statement was that every admissible subcategory induces a grothendieck topology on G, so we can extend the topology on G^{ad} by this topology in a a canonical way. The point is that you are not allowed to forget about G^{ad}, which provides the geometric structure (specifically, when defining algebraic stacks, we look at smooth morphisms in rather than étale morphisms. We do something similar with schemes, but schemes have a cover by smooth monomorphisms of representables, but smooth monics originating at affines are étale, so it suffices to say étale monic.)

    • CommentRowNumber15.
    • CommentAuthorUrs
    • CommentTimeApr 24th 2010
    • (edited Apr 24th 2010)

    Okay, I see what you mean. I have now rephrased the nLab entry at this point to make it clearer:

    An admissibility structure is

    • a choice of subcategory of admissible morphisms such that […]

    • a choice of coverage on VV by admissible covering morphisms.

      This choice of coverage, and not just the Grothendieck topology on VV that it generates, is part of the datum of the admissibility structure.

    Better?

    • CommentRowNumber16.
    • CommentAuthorHarry Gindi
    • CommentTimeApr 24th 2010

    The key point here is that the important data are G^{ad} and \tau such that \tau is generated by morphisms in G^{ad}. Remembering the coverage is unimportant so long as we know it can be generated by maps inside of G^{ad}. This is why I prefer saying that we have a grothendieck topology on the admissible subcategory itself. Since the admissible subcategory then generates its own topology, we can extend the topology on the subcategory to the whole category. This definition is less slick but intuitively easier to work with.

    • CommentRowNumber17.
    • CommentAuthorUrs
    • CommentTimeApr 24th 2010

    The key point here is that the important data are G adG^{ad} and τ\tau such that τ\tau is generated by morphisms in G adG^{ad}.

    Ahm, this is precisely what it said previously in the entry.

    All right, I’ll take now the time to sort this out, once and for all…

    • CommentRowNumber18.
    • CommentAuthorHarry Gindi
    • CommentTimeApr 24th 2010

    Yeah, but I'm still not really sure what you meant by this:

    I am wondering about this: except for the 2-out-of-3 condition, the definition of admissibility structure (in the second equivalent version that I put into the nLab entry) essentially just says: Grothendieck topology generated from a coverage.

    Which is not true. The admissibility structure is the pair 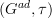(G^{ad},\tau), and in particular, we remember the subcategory as well. The fact that it's generated/extended by/from G^{ad} means precisely that our morphisms are compatible with the topology. This is much clearer if the topology is defined on the subcategory rather than generated in the subcategory.

    • CommentRowNumber19.
    • CommentAuthorUrs
    • CommentTimeApr 24th 2010

    Okay, I think we mean precisely the same thing (which is not too surprising, since I take we can both read what Lurie writes :-):

    I think a quick and helpful way to think of the definition is to say (now let’s see…):

    An admissibility structure is a Grothendieck topology with the special property that it is generated from a coverage whose morphisms come from a subcategory that satisfies a 2-out-of-3 condition.

    • CommentRowNumber20.
    • CommentAuthorHarry Gindi
    • CommentTimeApr 24th 2010
    • (edited Apr 24th 2010)

    No! That's the point that you keep missing! The admissibility structure is the subcategory AND the topology. This is similar, using the most basic example to how a smooth manifold is a set with a smooth structure, which is a topology and a sheaf of rings. Here, we have a category, a distinguished subcategory, and a topology on that subcategory. Explicitly, a geometric category in this sense is a triple (C,C^{ad},\tau) satisfying the axioms. What you're doing is equivalent to saying that a smooth structure on a set is only the topology. You're forgetting about more than half of the structure.

    • CommentRowNumber21.
    • CommentAuthorUrs
    • CommentTimeApr 24th 2010

    All right, it’s a structure, not a property. I don’t think I am missing that point, but you are right that somehow I fail to properly say it.

    • CommentRowNumber22.
    • CommentAuthorMike Shulman
    • CommentTimeApr 24th 2010

    So what is the practical import of the presence of the subcategory? How does it affect the definition of a “structure sheaf”?

    • CommentRowNumber23.
    • CommentAuthorUrs
    • CommentTimeApr 24th 2010
    • (edited Apr 24th 2010)

    So what is the practical import of the presence of the subcategory? How does it affect the definition of a “structure sheaf”?

    I don’t understand this well enough. Here is what i understand:

    the notion of admissible morphisms models the notion of open embedding or open immersions . The notion of admissibility structure serves to model the notion that among all possible covers we can single out the “open covers”.

    The structure sheaf is required only to respect these open covers, not any possibly more “singular” covers. So it is required to respect only the “coverage of open covers”. That’s the interpretation. I am not sure I can give a good account of what technically goes wrong if we reqired the structure 𝒪:𝒢→𝒳\mathcal{O} : \mathcal{G} \to \mathcal{X} to respect all covers. I suppose one gets the wrong notion of “locally ringed”.

    I need to have another look at Structured Spaces in order to make a better statement. Beyond this, all I have at this moment is the observation that when I was thinking about these “locally contractible oo-toposes” a similar condition played a central role: the claim is that oo-sheaves on a site form a locally contractible oo-topos if the site has two properties: 1) the covers are generated from good covers: the corresponding Cech nerve is a simplicial object internal to the site, and 2) with respect to that coverage all constant oo-stacks satisfy descent on all objects of the site. For instance CartSp becomes such a site if we take the generating covers to consist of precisely the ordinary good open covers.

    This can’t be entirely unrelated to what Lurie discusses. Notably his category of polynomial rings, discussed on page 81 as a motivation of pregeometries, is the algebraic analog of CartSp.

    Notice in this context, as I mentioned above, that David Spivak effectively worked with the “geometry” CartSp, but noticed that Lurie’s axioms don’t quite work here as expected. See page 61 here.

    • CommentRowNumber24.
    • CommentAuthorzskoda
    • CommentTimeApr 24th 2010
    • (edited Apr 24th 2010)

    Ad 3 Urs, Harry gave you link in Ad 1. Not the Barcelona.

    • CommentRowNumber25.
    • CommentAuthorHarry Gindi
    • CommentTimeApr 24th 2010
    • (edited Apr 24th 2010)

    The notion of admissible morphisms models the notion of open embedding or open immersions . The notion of admissibility structure serves to model the notion that among all possible covers we can single out the "open covers".

    The notion of an admissible morphism is more nuanced. Toën's notes use a slightly different formalism, which is, as I said, weaker, but the axioms can be proven if we're given an admissibility structure as in Lurie (and maybe a bit more, we may actually need a geometry, but that is pretty unimportant). If you want to get an intuitive understanding of what the admissible morphisms are, I suggest reading cours 1 and cours 2 of these notes:

    http://www.math.univ-toulouse.fr/~toen/m2.html

    • CommentRowNumber26.
    • CommentAuthorMike Shulman
    • CommentTimeApr 25th 2010

    The structure sheaf is required only to respect these open covers, not any possibly more “singular” covers.

    What I’m saying is that if a sheaf respects some coverage, then it also, automatically, respects all other covers generated from those. As long as “generated” means what I think it does, i.e. given a coverage, you take all sieves which contain a covering family in the given coverage. Thus, it seems that for purposes of defining a structure sheaf, it doesn’t matter whether you just know the Grothendieck topology, or whether you know some particular class of “good” covers from which it was generated.

    • CommentRowNumber27.
    • CommentAuthorHarry Gindi
    • CommentTimeApr 25th 2010
    • (edited Apr 25th 2010)

    This is true, but you use the morphisms in G^{ad} to classify the sheaves further. It's not just about satisfying descent. Being a stack on the étale site is weaker than being an algebraic stack because algebraic stacks have an atlas of smooth morphisms (where the term atlas has a specific meaning for stacks on a geometric site) in addition to satisfying étale descent (the point is that not every stack has this kind of atlas, which is composed of smooth morphisms, even though it is still a colimit of representables).

    The point that you're missing here is that we need more than just descent in geometry.

    • CommentRowNumber28.
    • CommentAuthorMike Shulman
    • CommentTimeApr 25th 2010

    Thanks, Harry, that’s what I was looking for. I alluded to my suspicion that that might be the answer back in comment #9, but it seems like everyone misunderstood what I was saying until now.

    I have to say that I think “admissibility structure” is a pretty useless name; it conveys absolutely no intuition to me. (-: Are there any alternatives in the literature?

    • CommentRowNumber29.
    • CommentAuthorHarry Gindi
    • CommentTimeApr 25th 2010
    • (edited Apr 25th 2010)

    I have to say that I think "admissibility structure" is a pretty useless name; it conveys absolutely no intuition to me.

    Quoted for truth. =) Luckily, it's not really in the category-theory canon, so we could come up with a better name for it.

    Edit: I guess Lurie didn't spend a week thinking of nothing else.

    (Yes, this was an appropriate time for that joke.)

    • CommentRowNumber30.
    • CommentAuthorMike Shulman
    • CommentTimeApr 25th 2010

    (Yes, this was an appropriate time for that joke.)

    Could be. Too bad you already ruined its humor value by making it three of four different times when it wasn’t as appropriate. (-:

    • CommentRowNumber31.
    • CommentAuthorUrs
    • CommentTimeApr 25th 2010
    • (edited Apr 25th 2010)

    The structure sheaf is required only to respect these open covers, not any possibly more “singular” covers.

    What I’m saying is that if a sheaf respects some coverage, then it also, automatically, respects all other covers generated from those.

    Wait, you are talking of something different here: the “structure sheaf” is a functor 𝒪:𝒢→𝒳\mathcal{O} : \mathcal{G} \to \mathcal{X}. For each fixed object g∈𝒢g \in \mathcal{G} it is an object of the oo-topos 𝒳\mathcal{X} and hence a “sheaf” on whatever 𝒳\mathcal{X} is the oo-topos of oo-sheaves on.

    But the structure sheaf functor 𝒪:𝒢→𝒳\mathcal{O} : \mathcal{G} \to \mathcal{X} is required to respect the admissible covers in 𝒢\mathcal{G} in the sense that if {U i→X}\{U_i \to X\} is a cover by admissible morphisms, then ∐ i𝒪(U i)→𝒪(X)\coprod_i \mathcal{O}(U_i) \to \mathcal{O}(X) is an effective epimorphism in 𝒳\mathcal{X}.

    • CommentRowNumber32.
    • CommentAuthorHarry Gindi
    • CommentTimeApr 25th 2010

    Could be. Too bad you already ruined its humor value by making it three of four different times when it wasn't as appropriate. (-:

    Things like that hurt the most when they're true =(

    • CommentRowNumber33.
    • CommentAuthorUrs
    • CommentTimeApr 25th 2010

    Mike, concerning what I wrote above:

    while true, I gather it still missses your point, sorry. I suppose that if ∐ i𝒪(U i)→𝒪(X)\coprod_i \mathcal{O}(U_i) \to \mathcal{O}(X) is an effective epimorphism for {U i→X}\{U_i \to X\} a covering, then also ∐ i𝒪(V i)→𝒪(X)\coprod_i \mathcal{O}(V_i) \to \mathcal{O}(X) will be an effective epimorphism, for {V i→X}\{V_i \to X\} the sieve generated from the covering.

    In fact, as remarked on the bottom of page 26 of StructuredSpaces, indeed the oo-category of 𝒢\mathcal{G}-structures on some 𝒳\mathcal{X} depends only on the Grothendieck topology on 𝒢\mathcal{G}, not on the admissibility structure. Rather, the purpose of the admissibility structure is to define the subcategory Str 𝒢(𝒳) loc⊂Str 𝒢(𝒳)Str^{loc}_{\mathcal{G}(\mathcal{X})} \subset Str_{\mathcal{G}}(\mathcal{X}) of 𝒢\mathcal{G}-structures and local morphisms between them. I started a new subsection on this:

    local morphisms of 𝒢\mathcal{G}-structures.

    • CommentRowNumber34.
    • CommentAuthorMike Shulman
    • CommentTimeApr 25th 2010

    Excellent! This is the sort of clarification I was looking for.

    If that’s the purpose of the admissibility structure, then maybe it should instead be called something like a “locality structure”?

    • CommentRowNumber35.
    • CommentAuthorHarry Gindi
    • CommentTimeApr 25th 2010
    • (edited Apr 26th 2010)

    Maybe Lurie was trying to avoid using the overloaded word "local" or something like that?

    Also, by the way, what I said and what Urs said translate to equivalent notions. If you view an oo-sheaf, oo-stack, etc., as the slice oo-topos living over it, what I said translates to what Urs says, and if you do the opposite, you get back what I said. It's just the application of the straightening or unstraightening functor to translate it.

    • CommentRowNumber36.
    • CommentAuthorUrs
    • CommentTimeApr 25th 2010

    If that’s the purpose of the admissibility structure, then maybe it should instead be called something like a “locality structure”?

    Yes, maybe.

    And we should further expand on this: because in fact the oo-category LTop(𝒢)LTop(\mathcal{G}) of oo-toposes with 𝒢\mathcal{G}-structure is (defined to be) such that the fiber over a fixed oo-topos 𝒳\mathcal{X} of the obvious projection LTop(𝒢)→LTopLTop(\mathcal{G}) \to LTop is Str 𝒢 loc(𝒳)Str^{loc}_{\mathcal{G}}(\mathcal{X}).

    I am trying to upload a section with this to the entry, but due to problems with my connection, maybe I haven’t succeeded yet (hard to tell for me, so bad is my connection…)

    • CommentRowNumber37.
    • CommentAuthorDavidRoberts
    • CommentTimeApr 26th 2010

    I’m going to be lazy here, and not read up the gazillion references you guys have thrown about. But in my thesis I have something called a class of admissible maps which I introduced to take care of things like submersions (but the final version my have changed since then, as I took Lie groupoids out of my thesis), when there was a handy pretopology/coverage lying around. It may or may not be useful here, even as just a metaphorical comparison. Let JJ be a fixed singleton pretopology and let EE be another singleton pretopology that contains JJ. Then EE is admissible for JJ if JJ is cofinal in EE and the following conditions hold:

    • The split epimorphisms are contained in EE,
    • If A→BA\to B is split epi and A→B→CA \to B \to C is in EE, then BtoCB to C is in EE.

    I have a feeling that this is a simple case of what is being discussed above, and may be a helpful test case.

    • CommentRowNumber38.
    • CommentAuthorMike Shulman
    • CommentTimeApr 26th 2010

    Thanks everyone. I am now wondering whether there is a “logical” incarnation of this. That is, a geometric theory can be presented in either a “syntactic” way with types, operations, and logical axioms, or in a more “extensional” way as a site, or in a maximally “extensional” way as a Grothendieck topos. So what is the syntactic counterpart of the extra “locality structure” on a site?

    • CommentRowNumber39.
    • CommentAuthorHarry Gindi
    • CommentTimeApr 26th 2010
    • (edited Apr 26th 2010)

    David, I believe that this agrees with Toën's definition for non-infinity-stuff (or is only slightly different). He introduced these admissible-class type thingies to deal with smooth morphisms, which are precisely the algebraic analogues of submersions. Unramified morphisms are the analogues of immersions, and étale morphisms are the analogues of local diffeomorphisms (local in the étale topology. The analogy fails in the Zariski topology (which is why Grothendieck invented grothendieck topologies in the first place!)).

    • CommentRowNumber40.
    • CommentAuthorDavidRoberts
    • CommentTimeApr 26th 2010

    @Harry,

    cool! It certainly is a pleasant coincidence that we came up with similar things and called them by similar names. For me it is interesting how the two classes interact when they are changed, and under what conditions they give rise to the same ’end result’. For example, we may want to maximise or make smaller the JJ (notation as in above comment) while keeping EE, or maximise EE relative to JJ and so on. (’maximise’ in the sense of being the largest such class of maps). For Urs’ benefit, I should point out that a canonical example of a class of admissible maps for the pretopology JJ is the topology it generates (with a little fiddling with the definition to take into account sieves, instead of singleton pretopologies).

    • CommentRowNumber41.
    • CommentAuthorHarry Gindi
    • CommentTimeApr 26th 2010

    @David, do you mean the union of all of the sieves in the grothendieck topology that the pretopology generates?

    • CommentRowNumber42.
    • CommentAuthorDavidRoberts
    • CommentTimeApr 26th 2010

    Well, I shouldn’t have said sieves. I meant the collection of all pullback-stable maps which admit local sections for the given pretopology JJ (I call it the saturation of JJ, but this is not standard as far as I am aware.) This is a singleton pretopology. Everything I wrote works for superextensive sites because all pretopologies can be replaced by equivalent singleton pretopologies, but I’m sure should work more generally. Sorry for the rushed message, but I’m going out soon (it’s a public holiday in Australia today)

    • CommentRowNumber43.
    • CommentAuthorHarry Gindi
    • CommentTimeApr 26th 2010
    • (edited Apr 26th 2010)

    I call it the saturation of J, but this is not standard as far as I am aware.

    Two pretopologies with the same saturation generate the same topology. The saturation is the supremum of a partial order that you can put on equivalent pretopologies (similar to how two atlases for a manifold generate the same structure sheaf if they have the same saturated atlas (maximal atlas) or how two filter-bases generate the same filter if they have the same maximal filter base), so your use of saturation is an abuse of terminology, but the good kind of abuse. Similarly, you can saturate any cover (covering family in a pretopology) to a sieve in a canonical way.

    • CommentRowNumber44.
    • CommentAuthorMike Shulman
    • CommentTimeApr 26th 2010

    I’m astonished that two people independently invented the same notion and managed to both call it by the same completely unintuitive (to me) name! David, can you explain why you chose “admissible”?

    • CommentRowNumber45.
    • CommentAuthorUrs
    • CommentTimeApr 26th 2010

    not read up the gazillion references you guys have thrown about.

    David, there are precisely only two references here, only: Lurie’s Structured Spaces and a set of lecture notes by Toen.

    • CommentRowNumber46.
    • CommentAuthorHarry Gindi
    • CommentTimeApr 26th 2010

    Well, and David Spivak's thesis.

    • CommentRowNumber47.
    • CommentAuthorUrs
    • CommentTimeApr 26th 2010

    For Urs’ benefit, I should point out that a canonical example of a class of admissible maps for the pretopology JJ is the topology it generates (with a little fiddling with the definition to take into account sieves, instead of singleton pretopologies).

    Thanks for pointing that out (why is it specifically for my benefit??), but if this is the case, then your use of admissible is pretty much opposite to the way Lurie uses it. He would call J the admissible covers for the topology it generates.

    Could you put in the details of what you do in your thesis on this matter into some nLab entry? If you can’t open the edit pages, post an entry here and I’ll copy it for you into the nLab.

    • CommentRowNumber48.
    • CommentAuthorUrs
    • CommentTimeApr 26th 2010

    So what is the syntactic counterpart of the extra “locality structure” on a site?

    To answer this, the first step should be to answer what the category of co-presheaves with values in a given topos means “syntactically”.

    I mean, this is what’s happening here: we have a category with a topology and want to consider both certain presheaves as well as certain co-presheaves on it, both respecting the topology in some way.

    And then for some reason we want to take not the full subcategory of co-presheaves that respect the topology, but a non-full subcategory. The locality structure is the extra datum on a topology that allows to find that subcategory of co-presheaves.

    Stated this way, does this remind you of anything that you have seen in logic?

    I expect what we need here is a bit of refined Isbell duality theory, or the like.

    • CommentRowNumber49.
    • CommentAuthorDavidRoberts
    • CommentTimeApr 26th 2010

    @Harry

    precisely only two references here

    Oh, I’ll just read a couple of the DAG’s and that should be fine :P Perhaps I should have said: ’the small number enormous references’. Anyway, I just wanted to throw in my two cents to see if it was helpful.

    Two pretopologies with the same saturation generate the same topology.

    Yeah, I was aware of this. I wanted to stay away from sieves in my thesis because I want topologists and differential geometers to (eventually) pick up the fine distinctions available using more than just the standard Grothendieck topology on Top or Diff (i.e. open covers or local homeo-/diffeomorphisms), especially for pathological spaces (non-paracompact and worse, like ’bad’ inf. dim manifolds). To me (a topologically minded person) talking about subfunctors of the yoneda embedding blah blah descent blah is not very intuitive. But saying: stuff glues together over these sort of maps (which I know is exactly the same thing :) which make up a singleton pretopology is less categorical machinery to learn.

    @Mike

    David, can you explain why you chose “admissible”?

    Originally I was just going with the class of maps that is the saturation of a singleton pretopology, but for a proof to work for Lie groupoids, I needed a more general class of maps (I didn’t think at the time that submersions formed the saturation of open covers, and in the end I didn’t need them to be). I called them ’admissible epis’ for a bit, but my notebook misses the actual day when I made up the name (presumably on some scrap piece of paper). Probably I used the name because they are admissible as a map to be the appropriate internalisation of ’surjective’ when saying ’essentially surjective functor’ so that the proof goes through. I vaguely recall writing a list of adjectives out of which I chose ’admissible’, and that name seemed to be the least overworked.

    @Urs,

    (why is it specifically for my benefit??)

    because that is the example you were quoting (even though the terminology is the other way around) and confusing the case that there was extra structure around: with the example of the saturation of a singleton pretopology, the saturation is unique, and so no extra data is needed, but in general there is needed something not supplied by just giving the coverage/pretopology.

    Could you put in the details of what you do in your thesis on this matter into some nLab entry? If you can’t open the edit pages, post an entry here and I’ll copy it for you into the nLab.

    See here for a little bit: you’re welcome to cut and paste into the lab - reference chapter 1 of my thesis. By the way, this will eventually become an paper on its own, so knowing about the stuff in this thread is good so I can reference Toen.

    • CommentRowNumber50.
    • CommentAuthorMike Shulman
    • CommentTimeApr 26th 2010

    I know what a copresheaf is syntactically—it’s a model of a theory. For instance, if C is a Lawvere theory, then a finite-product preserving copresheaf on C is a model of C (in whatever category). Likewise if T is a geometric theory, then its syntactic category Syn(T)Syn(T), aka its category of contexts, is a site with finite limits, and lex cover-preserving functors on Syn(T)Syn(T) are the same as models of T (in whatever category). And of course also equivalent to geometric morphisms into the classifying topos of T, which is the topos of sheaves on Syn(T)Syn(T).

    What I don’t yet understand is the syntactic meaning of this non-full-subcategory. What structure do we need to add to a geometric theory, say, so that Syn(T)Syn(T) becomes a geometry?

    • CommentRowNumber51.
    • CommentAuthorHarry Gindi
    • CommentTimeApr 26th 2010

    Oh, I'll just read a couple of the DAG's and that should be fine :P Perhaps I should have said: 'the small number enormous references'. Anyway, I just wanted to throw in my two cents to see if it was helpful.

    Why was that directed at me? Urs said that. =p

    • CommentRowNumber52.
    • CommentAuthorHarry Gindi
    • CommentTimeApr 26th 2010
    • (edited Apr 26th 2010)

    What I don't yet understand is the syntactic meaning of this non-full-subcategory. What structure do we need to add to a geometric theory, say, so that Syn(T) becomes a geometry?

    Mike, the fact that it's a subcategory is not really the important point. It's really just a class (set =p) of morphisms. When Lurie says that it's a subcategory, that's nothing more than saying that all identities (of the relevant objects [note: this is almost always a wide subcategory]) are in it, and it's closed under composition (I've also never seen a case where we don't require that it contains all isomorphisms (and therefore probably equivalences in the oo-case) as well).

    The other conditions let us put a local model structure on the category as well.

    I should note that in the ordinary algebraic case, the model structure is really cool. We look at stacks of groupoids and give it the following (cofibrantly generated) model structure:

    Cofibrations are (square-zero) nilpotent thickenings, weak equivalences are natural local weak equivalences of stacks of groupoids (what we actually mean by local is the only tricky part, but it's covered in Cours 8 of Toen's notes), and fibrations are smooth morphisms, where smooth morphisms are those that are (locally) finitely presented and have a (local) lifting property with respect to nilpotent thickenings (this is called formally smooth).

    Lurie's presentation is slick and excellent, but if you want to get another take on what exactly is going on, you should look at Toën and Vezzosi's HAG I and II, which carry out a similar approach to DAG using simplicially-enriched categories and model categories.

    • CommentRowNumber53.
    • CommentAuthorUrs
    • CommentTimeApr 26th 2010

    Mike, the fact that it’s a subcategory is not really the important point. It’s really just a class (set =p) of morphisms.

    We are talking about a different subcategory than you are thinking of here. We are talking about the category whose objects are cover-preserving functors 𝒪:𝒢→𝒳\mathcal{O} : \mathcal{G} \to \mathcal{X}, and whose morphisms are local transformations between these.

    • CommentRowNumber54.
    • CommentAuthorHarry Gindi
    • CommentTimeApr 26th 2010
    • (edited Apr 26th 2010)

    Nope, I'm pretty sure we're talking about the same thing. Look at my example from the algebraic case. You have to apply the (un?)straightening functor to get from what I'm saying to what you're saying. I mean, the example that I just gave is when the target category is groupoids. Lurie's approach is more general, but the same idea applies.

    • CommentRowNumber55.
    • CommentAuthorMike Shulman
    • CommentTimeApr 26th 2010

    Harry, are you saying that admissible morphisms are the same as local transformations?

    • CommentRowNumber56.
    • CommentAuthorHarry Gindi
    • CommentTimeApr 26th 2010
    • (edited Apr 27th 2010)

    No, here's the canonical example: Consider functors AlgSp^op -> Gpd, where the category of algebraic spaces is equipped with the following admissibility structure:

    Admissible morphisms are smooth.

    The grothendieck topology is just the étale topology on AlgSp.

    Now the thing is, we can now equip the functor category itself with an admissibility structure (at least in this case by the 2-Yoneda embedding and defining what a smooth (or etale) morphism of stacks is).

    Now, it's pretty obvious how we can generalize this to \infty-categories, at least if you read Toen's definition of what a local equivalence is (it's all phrased homotopically, so we can replace local equivalences of stacks of groupoids with local weak equivalences in our target oo-category). That's the brilliant thing about these notes: everything is phrased so we can swap groupoids for any simplicially-enriched category (in fact, for any homotopical category).

    The only tricky part of the whole affair is defining exactly what you mean by local.

    Now here's an example of a morphism of geometries: Consider the category of finite-type schemes over the complex numbers equipped with the restriction of the admissibility structure. We have a transformation of geometries by GAGA into the category of analytic complex manifolds. This is a transformation of geometries in the obvious way.

    I'll note that this is all covered in sections 3 and 4 of Structured Spaces in far more generality than I'm describing.

    In fact, what I'm describing to you is derived algebraic geometry, which is the title of section 4.3!

    Edit: I'd like to note something: What Lurie calls an etale morphism of rings is in fact what everyone else calls a smooth morphism of rings. He defines an etale morphism to be a morphism that is etale over a polynomial ring over a ring R, but this is a theorem of commutative algebra that all smooth R-algebras have such a presentation. I assume that he made this choice because he has used smooth to mean something else in the derived case. He even proves as a theorem that an etale morphism of simplicial commutative rings is a smooth morphism of discrete commutative rings.

    • CommentRowNumber57.
    • CommentAuthorUrs
    • CommentTimeApr 27th 2010
    • (edited Apr 27th 2010)

    back to #50, the question was:

    What structure do we need to add to a geometric theory, say, so that Syn(T)Syn(T) becomes a geometry?

    One other way to say it is this: you need a certain functorial assignment of factorization systems to the functor categories out of its classifying topos.

    This is an equivalent way of specifying the subcategory Str 𝒢 loc(𝒳)⊂Str 𝒢(𝒳)Str^{loc}_{\mathcal{G}}(\mathcal{X}) \subset Str_{\mathcal{G}}(\mathcal{X}) of 𝒢\mathcal{G}-structures on 𝒳\mathcal{X} and local transformations between them in terms of classifying oo-toposes:

    the point is that the local transformations are the right half of a factorization system on Str 𝒢(𝒳)Str_{\mathcal{G}}(\mathcal{X}), and that this factorization system depends functorially on 𝒳\mathcal{X}, in that for every geometric morphism 𝒳→𝒴\mathcal{X} \to \mathcal{Y} the induced Str 𝒢(𝒳)→Str 𝒢(𝒴)Str_{\mathcal{G}}(\mathcal{X}) \to Str_{\mathcal{G}}(\mathcal{Y}) respects these factorization systems. (theorem 1.3.1)

    This one can turn around, to characterize local transformations (and hence admissibility structures on 𝒢\mathcal{G}) in terms of functorial factorization systems on classifying oo-toposes (def. 1.4.3):

    For 𝒦\mathcal{K} an oo-topos, declare that a geometric structure on 𝒦\mathcal{K} is a choice of factorization systems on Topos geom(𝒳,𝒦) opTopos_{geom}(\mathcal{X}, \mathcal{K})^{op} that is functorial in 𝒳\mathcal{X} . Given such we have another way of saying “local transformation”: this is the non-full subcategory Str 𝒦 loc(𝒳)Str^{loc}_{\mathcal{K}}(\mathcal{X}) of Topos geom(𝒳,𝒦) opTopos_{geom}(\mathcal{X}, \mathcal{K})^{op} on all objects and on the right part of the factorization system.

    And this is indeed the same kind of datum as an admissibility structure on a geometry (example 1.4.4): in the case that 𝒦=Sh(𝒢)\mathcal{K} = Sh(\mathcal{G}) is the classifying topos for the geometry 𝒢\mathcal{G}, the defining equivalence Topos geom(𝒳,Sh(𝒢)) op→≃Str 𝒢(𝒳)Topos_{geom}(\mathcal{X}, Sh(\mathcal{G}))^{op} \stackrel{\simeq}{\to} Str_{\mathcal{G}}(\mathcal{X}) identifies the two sub-categories of local transformations, Str 𝒢 loc(𝒳)Str^{loc}_{\mathcal{G}}(\mathcal{X}) and Str Sh(𝒢) loc(𝒳)Str^{loc}_{Sh(\mathcal{G})}(\mathcal{X}).

    • CommentRowNumber58.
    • CommentAuthorDavidRoberts
    • CommentTimeApr 27th 2010

    @Harry

    Why was that directed at me?

    whoops, so he did.

    @Mike

    further to my comment about nomenclature, the intent was to consider ambient categories (for the purposes of internal groupoids) that were not finitely complete, and the admissible maps were to be the class of maps from which the source and target maps were to be drawn, much as the source and target maps for a Lie groupoid are generally (but not of necessity) specified to be submersions (a postiori surjective, as they are split maps).

    • CommentRowNumber59.
    • CommentAuthorMike Shulman
    • CommentTimeApr 27th 2010

    Okay, Harry, if the answer to #55 is “no,” then I think Urs was right in #53. The admissibility structure is a class of morphisms in the category GG, but we were talking about the category of GG-structures and local morphisms between them.

    The factorization system point of view is helpful. Also, I just recalled that there is a quite similar characterization of elementary embeddings between logical structures. In fact, it seems to me at first glance that the syntactic category of a geometric theory should come with an admissibility structure consisting of all monomorphisms, such that the “local morphisms” of models are precisely the elementary embeddings. If that’s right, then just as an elementary embedding is one that “preserves and reflects truth of all logical constructions,” it would make sense to say that a local morphism is one that “preserves and reflects a specified class of (not necessarily purely logical) constructions.”

    • CommentRowNumber60.
    • CommentAuthorHarry Gindi
    • CommentTimeApr 27th 2010
    • (edited Apr 27th 2010)

    I guess that the case I was thinking of was a special case since we can extend the admissibility structure by embedding G in the subcategory of stacks in [G^op, Gpd].

    Can we always embed G in Str_G(X)? Can we do it if the topology induced on G is subcanonical?

    • CommentRowNumber61.
    • CommentAuthorUrs
    • CommentTimeApr 27th 2010
    • (edited Apr 27th 2010)

    Can we always embed \mathcal{G} in $Str_G(X)? Can we do it if the topology induced on G is subcanonical?

    No. In the special case that 𝒳\mathcal{X} happens to be 𝒳=∞Grpd\mathcal{X} = \infty Grpd you might have a chance of embedding the opposite 𝒢 op\mathcal{G}^{op} into Str 𝒢(𝒳=∞Grpd)Str_{\mathcal{G}}(\mathcal{X} = \infty Grpd). It works at least if the very special case that the topology on 𝒢\mathcal{G} is trivial/discrete, because then that’s the Yoneda embedding. Even if the topology on 𝒢\mathcal{G} is more general but subcanonical I don’t see that you get a factorization, because the conditions satified by objects in Str 𝒢(∞Grpd)Str_{\mathcal{G}}(\infty Grpd) are not those of sheaves/oo-stacks on 𝒢 op\mathcal{G}^{op}, and even if they were, we are not talking about topologies on 𝒢 op\mathcal{G}^{op} but on 𝒢\mathcal{G}.

    To recall: Str 𝒢(𝒳)Str_{\mathcal{G}}(\mathcal{X}) is the category whose objects are finite limit preserving functors 𝒪:𝒢→𝒳\mathcal{O} : \mathcal{G} \to \mathcal{X} (co-presheaves on 𝒢\mathcal{G}!) that send covering families to effective epimorphisms in the (∞,1)(\infty,1)-topos 𝒳\mathcal{X}. And the discussion since #33 and then more forcefully since #50 is about the question how we are to think of the non-full subcategory Str 𝒢 loc(𝒳)⊂Str 𝒢(𝒳)Str^{loc}_{\mathcal{G}}(\mathcal{X}) \subset Str_{\mathcal{G}}(\mathcal{X}) inside there, whose morphisms are only those transformations that respect the admissibility structure on 𝒢\mathcal{G} (in that their naturality squares on admissible morphisms are required to be pullback squares).

    This is the only role that the admissibility structure plays in the definition of the category of 𝒢\mathcal{G}-structured (∞,1)(\infty,1)-toposes, and so we are trying to understand if we can recognize this restriction to Str 𝒢 loc(𝒳)Str^{loc}_{\mathcal{G}}(\mathcal{X}) as an operation familiar in other terms in topos theory.

    • CommentRowNumber62.
    • CommentAuthorDavidRoberts
    • CommentTimeApr 27th 2010

    Some rushed thoughts:

    finite limit preserving functors…that send covering families to effective epimorphisms

    so we could say that it is a map of sites? Where 𝒳\mathcal{X} has the effective epimorphism pretopology/coverage (I think some rejigging needs to be done, since a non-singleton covering family can’t be sent to a single map, but the details can be thrashed out later).

    Since transformations are functors G→Arr(X)G \to Arr(X) (I’m being slack here, and only thinking of the 1-cat case, which is all I can claim to really understand), can we say that there is some sort of structure on Arr(X)Arr(X) like a pretopology, such that transformations G→Arr(X)G \to Arr(X) are also maps of sites, where now GG is taken to be a site with the admissibility structure as the pretopology? Or maybe we can talk about lifts of functors G→XG \to X up the fibrations Arr(X)→XArr(X) \to X (or the putative fibration Arr(X)→X×XArr(X) \to X\times X) so that the components of the lifts are cartesian arrows (hence pullback squares)?

    • CommentRowNumber63.
    • CommentAuthorUrs
    • CommentTimeApr 27th 2010

    I just recalled that there is a quite similar characterization of elementary embeddings between logical structures.

    Thanks, that looks interesting. I dropped you a query box at elementary embedding, where I am just checking if I am precisely following.

    • CommentRowNumber64.
    • CommentAuthorUrs
    • CommentTimeApr 27th 2010
    • (edited Apr 27th 2010)

    Since transformations are functors G→Arr(X)G \to Arr(X) […], can we say that there is some sort of structure on Arr(X)Arr(X) like a pretopology, such that transformations G→Arr(X)G \to Arr(X) are also maps of sites, where now GG is taken to be a site with the admissibility structure as the pretopology?

    I see what you mean. You could declare pullback squares in 𝒳\mathcal{X} to be the “admissible” morphisms in Arr(𝒳)Arr(\mathcal{X}). Indeed, this will equip Arr(𝒳)Arr(\mathcal{X}) with an admissibility structure: the required 2-out-of-3-property is the pasting law for pullback squares! Then the local transformations η:𝒪⇒𝒪′:𝒢→𝒳\eta : \mathcal{O} \Rightarrow \mathcal{O}' : \mathcal{G} \to \mathcal{X} would be those natural transformations whose components 𝒢→Arr(𝒳)\mathcal{G} \to Arr(\mathcal{X}) respect admissible morphisms. Yes.

    • CommentRowNumber65.
    • CommentAuthorHarry Gindi
    • CommentTimeApr 27th 2010
    • (edited Apr 27th 2010)

    This is the only role that the admissibility structure plays in the definition of the category of G-structured (oo,1)-toposes, and so we are trying to understand if...

    Gah, Lurie's formalism is completely different and confusing. He emphasizes the view of a geometric object as a locally ringed topos, while Toen-Vezzosi (in HAG) treat geometric objects as functors of points. We are actually talking about the same thing, but I'm not familiar enough with Lurie's formalism or the stuff in HTT to continue commenting. I appreciate your patience and find this discussion stimulating =).

    I really need to get through HTT, but the foundational material is really a pain, and I can't stand reading books out of order =(. (I haven't made any progress in ch.2 since christmas. I got up to 2.1.4 and haven't been able to give it serious amounts of time since then.)

    If anyone here (I know, I'm reaching here, since all of you guys probably think that it's child's play) hasn't read through chapters 2-4 of HTT yet (or only glanced at them) and is interested to actually read them, I'd appreciate it if we could somehow correspond through e-mail and discuss it. (I'm not asking anyone who has read it, because I don't want to waste your time!)

    When I read books alone, I get caught up on stupid things like "is that a mistake or am I misunderstanding the argument?" or "am I understanding the argument wrong, or is this little bit of information unimportant to the proof?" (I'm specifically thinking about when Lurie notes in the last proof of 2.1.3 in the last paragraph of the first page of the proof that X is a kan complex, while it doesn't appear that it's at all relevant.), so it really helps me to have someone to whom I can pose a quick question like that.

    • CommentRowNumber66.
    • CommentAuthorUrs
    • CommentTimeApr 27th 2010
    • (edited Apr 27th 2010)

    He emphasizes the view of a geometric object as a locally ringed topos, while Toen-Vezzosi (in HAG) treat geometric objects as functors of points.

    Not really. Lurie has the full picture. We just happen to be discussing the special cases of ringed toposes / structured toposes here in this discussion.

    I once tried to summarize the big picture that Structured Spaces establishes in that second standout box at Notions of space.

    The story is this:

    given a geometry 𝒢\mathcal{G} (in the sense we are discussing, i.e. oo-site with admissibility structure), we may think (as you know, but let me just repeat it for emphasis) of the objects in 𝒢\mathcal{G} as test spaces, and as the objects in H=Sh (∞,1)(𝒢)\mathbf{H} = Sh_{(\infty,1)}(\mathcal{G}) as “very general spaces modeled on 𝒢\mathcal{G}”.

    Toen-Vezzosi’s work is about giving model-category models for this Sh (∞,1)(𝒢)Sh_{(\infty,1)}(\mathcal{G}) for the case that 𝒢\mathcal{G} is not just an ordinary category, but a genuine (∞,1)(\infty,1)-category (where previously Brown, Joyal, Jardine, Dugger, et al had concentrated on the case of ∞\infty-stacks on just ordinary 1-categorical sites): it is their model structure on sSet-enriched presheaves. (Of course Lurie in HTT shows that this model is indeed a presentation of Sh (∞,1)(𝒢)Sh_{(\infty,1)}(\mathcal{G}).

    But now comes the point: in between the two extremes, the very specific but very tame test spaces 𝒢\mathcal{G} and the very general but possibly quite “wild” spaces Sh (∞,1)(𝒢)Sh_{(\infty,1)}(\mathcal{G}) modeled on them, one can find notions of generalized spaces that are not too wild. One of that is a 𝒢\mathcal{G}-scheme (as you know): an object in Sh (∞,1)(𝒢)Sh_{(\infty,1)}(\mathcal{G}) that, while not representable, is locally representable i.e. locally equivalent to a test space in 𝒢\mathcal{G}.

    Here in this discussion, we have been concentrating on something more general than 𝒢\mathcal{G}-schemes: the 𝒢\mathcal{G}-structured (∞,1)(\infty,1)-toposes. Notice that, as Lurie shows, these can also be regarded as sitting inside Sh (∞,1)(𝒢)Sh_{(\infty,1)}(\mathcal{G}). But they have the special property that, while not necessarilly locally representable, they are at least concrete in the sense of concrete sheaf: they do have an underlying topological space – or at least an underlying (∞,1)(\infty,1)-topos 𝒳\mathcal{X}, to be regarded as a generalized topological space.

    𝒢↪Schemes(𝒢)↪𝒢−structuredToposes↪Sh (∞,1)(𝒢). \mathcal{G} \hookrightarrow Schemes(\mathcal{G}) \hookrightarrow \mathcal{G}-structured\;Toposes \hookrightarrow Sh_{(\infty,1)}(\mathcal{G}) \,.

    Notice that (∞,1)(\infty,1)-toposes appear in two different roles here: as gros topos and as petit topos: the big huge (∞,1)(\infty,1)-topos H=Sh (∞,1)(𝒢)\mathbf{H} = Sh_{(\infty,1)}(\mathcal{G}) is the collection of “all spaces modeled on 𝒢\mathcal{G}”. On the other hand the underlying (∞,1)(\infty,1)-toposes 𝒳\mathcal{X} of 𝒢\mathcal{G}-structured (∞,1)(\infty,1)-toposes are petit: they are really to be thought of as sheaves on just a single topological space. They all sit inside the big H\mathbf{H} as objects.

    (I am glossing intentionally over some size issues.)

    It’s a grand story. It may be helpful to think of it in terms of the following simple 1-categorical example: consider the site 𝒢=\mathcal{G} = CartSp. Then

    • a representable is a Cartesian space;

    • a locally representable sheaf on CartSp is a manifold;

    • a topological space with CartSpCartSp-valued structure sheaf is a Frölicher space;

    • a general sheaf on CartSp is … well, whatever it is.

    So here in this case the above sequence of inclusions of tame into incresingly wild spaces modeled on the geometry 𝒢\mathcal{G} is

    CartesianSpaces↪Manifolds↪FroelicherSpaces↪Sh(CartSp). CartesianSpaces \hookrightarrow Manifolds \hookrightarrow FroelicherSpaces \hookrightarrow Sh(CartSp) \,.
    • CommentRowNumber67.
    • CommentAuthorHarry Gindi
    • CommentTimeApr 27th 2010
    • (edited Apr 27th 2010)

    Alright, so I wasn't as totally off the mark as I thought, which is somewhat encouraging. Anyway, I don't think that I have anything more to add to the discussion (if one could even say that I added anything =). I'm not sure if what I was saying was totally useless or at least partially on the mark).

    (I am glossing intentionally over some size issues.)

    Especially useful given my obstinate stand regarding size issues!

    Anyway, regarding what I said about corresponding with someone reading HTT chapters 2-4, if any of you guys have students doing this, I would love to correspond with them as well (again, I realize, this may be at best wishful thinking!). I'm looking for a teammate (or someone to do an informal reading course type of thing with, at least), so if you know anyone who might be interested in corresponding through e-mail while reading through it, I'm just putting it out there (my grandfather has told me that you'll never get anything if you don't ask.)

    • CommentRowNumber68.
    • CommentAuthorUrs
    • CommentTimeApr 27th 2010

    Anyway, regarding what I said about corresponding with someone reading HTT chapters 2-4, if any of you guys have students doing this, I would love to correspond with them as well (again, I realize, this may be at best wishful thinking!). I’m looking for a teammate (or someone to do an informal reading course type of thing with, at least), so if you know anyone who might be interested in corresponding through e-mail while reading through it,

    As I suppose you have heard me say, in Utrecht we are currently running a seminar going through HTT. You have seen me add material to nLab entries as we went along, in parts this was material supplementing talks thatmembers of our group gave in our Friday seminar. The last things we did was stuff related to limit in a quasi-category and adjoint (infinity,1)-functors. (All this is still very imperfect, as time is short and HTT is long. :-) So far our group members have been looking at the nLab, but not quite went to the point of interacting here. But one thing you could do – and I would be quite glad if you did – would be to drop query boxes all over the place in the HTT-related entries on the nLab. Then I could point people to it and maybe get some more interaction started.

    I’m just putting it out there (my grandfather has told me that you’ll never get anything if you don’t ask.)

    I like that advice.

    • CommentRowNumber69.
    • CommentAuthorHarry Gindi
    • CommentTimeApr 27th 2010
    • (edited Apr 27th 2010)

    As I suppose you have heard me say, in Utrecht we are currently running a seminar going through HTT.

    Actually, this is news to me! It's somewhat unfortunate that I didn't hear about this when you started it!

    What specific part of the book (chapter/section) are you up to?

    • CommentRowNumber70.
    • CommentAuthorUrs
    • CommentTimeApr 27th 2010

    We are following roughly the plan indicated here, where the last thing we did so far is the bit about adjoint oo-functors. So in the book we are now pretty much exactly at after section 5.2.

    But I should say that we are kind of “zooming in” to the material instead of developing every little detail. The plan is that at the end of the semester, which is in a few weeks, those who followed the seminar will understand the concept of a left exact accessible localization of an oo-presheaf category – hence of an oo-topos, and know some of its basic properties.

    So later this week I’ll start filling in more material on locally presentable and accessible oo-categories into the nLab and on localizations. Then in two weeks we start talking about the exact localizations, and I’ll be filling in more details on that.

    • CommentRowNumber71.
    • CommentAuthorMike Shulman
    • CommentTimeApr 27th 2010

    Hmm, my #59 is not quite right, since elementary embeddings are usually about all (finitary) first-order formulas, which don’t necessarily exist in the syntactic category of a geometric theory. But it would be if instead we meant “geometrically elementary” embeddings.

    • CommentRowNumber72.
    • CommentAuthorUrs
    • CommentTimeApr 27th 2010

    But it would be if instead we meant “geometrically elementary” embeddings.

    Ahm, sorry. This sounds very interesting, but I am not sure anymore what exactly you have in mind. Could you spell this out in full detail in the entry on elementary embeddings?

    • CommentRowNumber73.
    • CommentAuthorMike Shulman
    • CommentTimeApr 27th 2010

    Yeah, I will when I get a moment.

1 to 73 of 73

  1.