nForum
Not signed in
Want to take part in these discussions? Sign in if you have an account, or apply for one below
Site Tag Cloud
Vanilla 1.1.10 is a product of Lussumo. More Information: Documentation, Community Support.
If you want to take part in these discussions either sign in now (if you have an account), apply for one now (if you don't).
-
- CommentRowNumber1.
- CommentAuthorAndrew Stacey
- CommentTimeFeb 3rd 2010
Through completely my own fault, I find myself having agreed to give a colloquium level talk on category theory and having not enough time to properly prepare it. So I thought I'd throw myself on the mercy of the nCrew and see if someone could help me out a little. In short, I need two things:
A snazzy diagram about category theory to go with the announcement (no need to draw it, just an idea). So nothing technical, or too complicated, but can be slightly silly (i.e. a serious diagram that nonetheless makes a silly shape).
A "killer app" for category theory. To expand on that, I'm not going to assume too much sophistication on behalf of my audience and most of them are on the applied side (numerics, operator algebras) so I don't intend getting too technical. My vague idea is to explain some of the basic ideas with illustrations that they'll be fairly familiar with but making connections that they're not so familiar with. So the fact that Banach spaces are more like groups than they are like normed vector spaces is something I'll (probably) say something about. But I need a "punchline" to finish with. Something to round things off and leave everyone thinking "Wow, that's kinda cool".
I can do the pizzazz and tell a good story (well, I think I can) but being relative newcomer to category theory then I'm aware that I don't know all the ins and outs and the Big Ideas. Plus, as I said, I'm running out of time (where's The Doctor when you need him? He's been seen in Norway once or twice.). So any ideas very gratefully received!
-
- CommentRowNumber2.
- CommentAuthorHarry Gindi
- CommentTimeFeb 3rd 2010
- (edited Feb 3rd 2010)
I've always found Yoneda to be the most impressive single statement of category theory, and it's quite easy to prove. You can talk about the Riesz representation theorem, and then show them the yoneda lemma. You can talk about the Day convolution, yoneda reduction, and all of that good wholesome stuff. -
- CommentRowNumber3.
- CommentAuthordomenico_fiorenza
- CommentTimeFeb 3rd 2010
my vote for yoneda, too. something like "(pre)sheves are generalized objects exactly the same way distributions are genralized functions". and the functor of points I find is a nice example of this: in many situations people in analysis use it without knowing (essentially all times they work with spaces of maps) -
- CommentRowNumber4.
- CommentAuthorUrs
- CommentTimeFeb 3rd 2010
- (edited Feb 3rd 2010)
concering killer app:
a large class of killer apps will be of the form: thisandthat familiar entity is really an object in thisandthat topos.
Once we have it realized there in the topos, then playing around with hom adjunctions reproduces bunches of otherwise more mysetrious statements in a very elegant and transparent way.
That also involves the central magic boxes that category theory is about: adjunctions first of all, and maybe presheaves, if you want to go into that.
For instance, take what I just wrote at differential equation.
All we need is to assume that we have a category that is cartesian closed, whose objects we want to think of as smooth spaces. And that there is one object D such that homming it into any other object produces the tangent bundle

Depending on how one wants to present this, one can take this as the defining property or go a bit further into how to construct such D, which also involves nice category theoretic tools.
Once we have this, and just this, lots of traditionally quite sophisticated sounding statements become tautologies.
For instance: prove that a vector field is the tangent to a path of diffeomorphisms
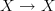 starting at the identity map: with our category theory this is now just the hom-adjunction in the cartesian closed category:
starting at the identity map: with our category theory this is now just the hom-adjunction in the cartesian closed category: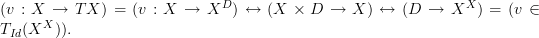
That's pretty cool, if one thinks about it. It is important here, of course, not to forget over the triviality of these category theoretic manipulations the sound of this statement in traditional language.
I think one can list a bunch of examples of this sort. So if you like that, I can try to go on. But I'll stop here to hear what you think.
-
- CommentRowNumber5.
- CommentAuthorAndrew Stacey
- CommentTimeFeb 3rd 2010
Yes, I figured that the idea of testing something by throwing simple things at it would go down well with functional analysts. Somehow, though, I'm having a mental block on seeing a simple application of Yoneda directly in functional analysis (but it's late here so that may just be brain going into shutdown mode).
-
- CommentRowNumber6.
- CommentAuthorAndrew Stacey
- CommentTimeFeb 3rd 2010
(Oops, cross-posted with Urs there.)
I did notice that you just added something about differential equations in a smooth topos. That looks very interesting. But as I said in my post just above, my brain's about to shutdown for the night so I'll have a think about what you wrote tomorrow and get back to you.
(I certainly don't want anyone to do the hard slog for me on this.)
-
- CommentRowNumber7.
- CommentAuthorUrs
- CommentTimeFeb 3rd 2010
Vaughan Pratt has been advertizing his article The Yoneda lemma without category theory: algebra and applications as being a source of lots of examples of applicatins of the lemma. His applications are of course not on the functional analytic side of life, but in algebra. But maybe you should browse throught it and see what you can find.
-
- CommentRowNumber8.
- CommentAuthorTodd_Trimble
- CommentTimeFeb 4th 2010
- (edited Feb 4th 2010)
Andrew, I'm not strongly suggesting you use this, but I recall that I once gave a colloquium talk on a little app of categorical thinking titled "On a problem of Halmos". In his automathography, Halmos points with pride to a final exam he gave for a course on topological groups, consisting of 15 open-ended questions ("geniuses are expected to solve all fifteen"); one of the questions was "does there exist a connected topological group of exponent 2?" (For him, all topological groups are by default Hausdorff.)
I started by throwing the audience off the scent, suggesting that the answer might be "no" if we think of topological groups of exponent 2 as "TVS" over the discrete field Z mod 2 and if we expect to be able to prove a Hahn-Banach theorem that there exist nontrivial continuous functionals. But the answer is "yes", and this can be seen easily by thinking in terms of algebraic theories. The idea is that groups in which
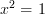 for all
for all  is interpretable in any category with finite products, and that product-preserving functors
is interpretable in any category with finite products, and that product-preserving functors  always map such a structure in
always map such a structure in  to such a structure in
to such a structure in  . Then I produced a series of product-preserving functors
. Then I produced a series of product-preserving functors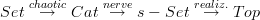
which takes the structure
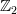 in
in 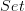 over to the total space
over to the total space 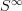 of the classifying bundle of
of the classifying bundle of 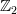 , as a structure of this type in
, as a structure of this type in 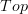 . This structure is connected, and: we're done! Simple. It was a leisurely-paced talk and went over well with the functional analysts in the crowd.
. This structure is connected, and: we're done! Simple. It was a leisurely-paced talk and went over well with the functional analysts in the crowd. -
- CommentRowNumber9.
- CommentAuthorAndrew Stacey
- CommentTimeFeb 6th 2010
I'm beginning to get a feel for how I want the seminar to go. The colloquium series here goes by the name of "Mathematical Pearls". My abstract can be found here (none of you suggested a diagram so I had to invent something suitably daft!). My current sketch plan is:
First, the disclaimer. This isn't history and I'm no expert. This is more "how it might have been".
First irritating thing: you can't talk about "the set of all sets". EM wanted to do so to talk about cohomology theories and specifically operations on cohomology theories. So they invented "categories" to allow them to do so. The "deal with Russell" (i.e. avoiding the paradox) was that they would steer clear of anything that might bring them close to the paradox. This leads one naturally to avoid examining objects too closely and concentrating on morphisms.
Second irritating thing: group-like things crop up everywhere, such as in homotopy groups, and it's annoying to have to keep proving the same things over and over again. If we could only have a "platonic group" for which we could prove stuff once and for all, we wouldn't have to keep doing it over and over again. Lawvere gave us such, and it means that we can use Eckman-Hilton to prove that
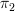 is abelian rather than the complicated shuffle homotopy. (I quite like that shuffle, but I won't say so!)
is abelian rather than the complicated shuffle homotopy. (I quite like that shuffle, but I won't say so!)So we can talk about "group objects" in (almost) any category. But clearly, there's nothing special about groups. We can do the same for rings, modules, monoids, Banach spaces ... huh? Banach spaces??? How did they creep in there? Then explain that Banach spaces are (almost) algebraic and that
 -algebras actually are.
-algebras actually are.There's categories everywhere. Once you have "categorical glasses" on then you can't help seeing categories anywhere and everywhere. Groups, posets, lattices, metric spaces ... huh?
But I still feel that I'm missing something for the ending. I agree that Yoneda is extremely important, and I like the recasting of differential equations in categorical form, but of the above (and I haven't looked at Vaughan's list yet), Todd's is the closest to what I want. Describing or recasting isn't quite the dramatic conclusion that I'm after. I'll take a look at Vaughan's list to see if anything jumps out at me.
Incidentally, there'll be a fair few algebra people there as well.
-
- CommentRowNumber10.
- CommentAuthorHarry Gindi
- CommentTimeFeb 6th 2010
Any chance you could film it and put it on the internet? I'd be interested in watching it. -
- CommentRowNumber11.
- CommentAuthorAndrew Stacey
- CommentTimeFeb 12th 2010
In case anyone's interested, the slides of this talk are now available at my seminar page. Sorry, wasn't able to video/record it.
Please, please, please can I link my video from Eckmann-Hilton!
(Though I can see that some might think I've taken the idea of "proof without words" just that one step too far)
1 to 11 of 11