nForum
Not signed in
Want to take part in these discussions? Sign in if you have an account, or apply for one below
Site Tag Cloud
Vanilla 1.1.10 is a product of Lussumo. More Information: Documentation, Community Support.
If you want to take part in these discussions either sign in now (if you have an account), apply for one now (if you don't).
-
- CommentRowNumber1.
- CommentAuthorUrs
- CommentTimeMar 15th 2010
- (edited Mar 15th 2010)
The nCafé is currently haunted by a bug that prevents any comments from being posted. This should eventually go away, hopefully. For the time being I post my comment in reply to the entry Division Algebras and Supersymmetry II here:
Thanks, John and John for these results. This is very pleasing.
The 3-
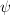 s rule implies that the Poincaré superalgebra has a nontrivial 3-cocycle when spacetime has dimension 3, 4, 6, or 10.
s rule implies that the Poincaré superalgebra has a nontrivial 3-cocycle when spacetime has dimension 3, 4, 6, or 10.Similarly, the 4-
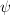 s rule implies that the Poincaré superalgebra has a nontrivial 4-cocycle when spacetime has dimension 4, 5, 7, or 11.
s rule implies that the Poincaré superalgebra has a nontrivial 4-cocycle when spacetime has dimension 4, 5, 7, or 11.Very nice! That's what one would have hoped for.
Can you maybe see aspects of what makes these cocycles special compared to other cocycles that the Poincaré super Lie algebra has? What other cocycles that involve the spinors are there? Maybe there are a bunch of generic cocycles and then some special ones that depend on the dimension?
Is there any indication from the math to which extent
 and
and 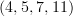 are the first two steps in a longer sequence of sequences? I might expect another sequence
are the first two steps in a longer sequence of sequences? I might expect another sequence 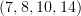 and
and 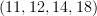 corresponding to the fivebrane and the ninebrane. In other words, what
happens when you look at
corresponding to the fivebrane and the ninebrane. In other words, what
happens when you look at  -matrices with values in a division algebra for values of
-matrices with values in a division algebra for values of
 larger than 2 and 4?
larger than 2 and 4?Here a general comment related to the short exact sequences of higher Lie algebras that you mention:
properly speaking what matters is that these sequences are
 -categorical exact, namely are fibration sequences/fiber sequences in an
-categorical exact, namely are fibration sequences/fiber sequences in an  -category of
-category of  -algebras.
-algebras.The cocycle itself is a morphism of
 -algebras
-algebras
and the extension it classifies is the homotopy fiber of this
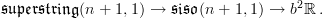
Forming in turn the homotopy fiber of that extension yields the loop space object of
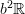 and thereby the fibration sequence
and thereby the fibration sequence
The fact that using the evident representatives of the equivalence classes of these objects the first three terms here also form an exact sequence of chain complexes is conceptually really a coicidence of little intrinsic meaning.
One way to demonstrate that we really have an
 -exact sequence here is
to declare that the
-exact sequence here is
to declare that the  -category of
-category of 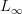 -algebras is
that presented by the standard modelstructure on dg-algebras on
-algebras is
that presented by the standard modelstructure on dg-algebras on  . In there
we can show that
. In there
we can show that  is
homotopy exact by observing that this is almost a fibrant diagram, in that
the second morphism is a fibration, the first object is fibrant and the
other two objects are almost fibrant: their Chevalley-Eilenberg algebras are
almost Sullivan algebras in that they are quasi-free. The only failure of
fibrancy is that they don't obey the filtration property. But one can pass
to a weakly equivalent fibrant replacement for
is
homotopy exact by observing that this is almost a fibrant diagram, in that
the second morphism is a fibration, the first object is fibrant and the
other two objects are almost fibrant: their Chevalley-Eilenberg algebras are
almost Sullivan algebras in that they are quasi-free. The only failure of
fibrancy is that they don't obey the filtration property. But one can pass
to a weakly equivalent fibrant replacement for 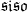 and do the
analog for
and do the
analog for 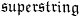 without really changing the nature of the
problem, given how simple
without really changing the nature of the
problem, given how simple 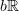 is. Then we see that the sequence is
indeed also homotopy-exact.
is. Then we see that the sequence is
indeed also homotopy-exact.This kind of discussion may not be relevant for the purposes of your article, but it does become relevant when one starts doing for instance higher gauge theory with these objects.
Here some further trivial comments on the article:
Might it be a good idea to mention the name "Fierz" somewhere?
page 3, below the first displayed math: The superstring Lie 2-superalgebra is [an] extension of
p. 4: the bracket of spinors defines [a] Lie superalgebra structure
p. 6, almost last line: this [is] equivalent to the fact
p. 13 this spinor identity also play[s] an important role in
p. 14: recall this [is] the component of the vector
-
- CommentRowNumber2.
- CommentAuthorDavid_Corfield
- CommentTimeMar 15th 2010
- (edited Mar 15th 2010)
Is there any indication from the math to which extent (3,4,6,10) and (4,5,7,11) are the first two steps in a longer sequence of sequences? I might expect another sequence (7,8,10,14) and (11, 12, 14, 18) corresponding to the fivebrane and the ninebrane.
Aren't the first two sequences supposed to be from the columns in Duff's chart in the post? Is the specialness of 5 and 9 connected to their being (1+4) and (1+8)?
-
- CommentRowNumber3.
- CommentAuthorUrs
- CommentTimeMar 15th 2010
Is the specialness of 5 and 9 connected to their being (1+4) and (1+8)?
Well, at least one has to be careful with the numerology here, as the string/=1-brane and membrane=2-brane would not fit that pattern that you suggest.
But I think there is yet another pattern running here, where "fundamental n-branes" exists for
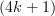 (string, fivebrane, ninebrane) whose worldvolume theory is conformal, and then one dimension higher runs the sequence of
(string, fivebrane, ninebrane) whose worldvolume theory is conformal, and then one dimension higher runs the sequence of 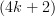 -branes whose worldvolume theory is the corresponding Chern-Simons theory (membranes, etc.).
-branes whose worldvolume theory is the corresponding Chern-Simons theory (membranes, etc.).But I have only a vague understanding of the general pattern here.
-
- CommentRowNumber4.
- CommentAuthorJohn Baez
- CommentTimeMar 15th 2010
<div> Thanks for all your comments! I'm really bummed out that we need to have this discussion here instead of on the n-Cafe, due to technical problems. I want to get people interested in John Huerta's work so he gets a job! Sometime when I can post comments to the n-Cafe I'm going to copy your comments over there.<br/><br/>Anyway:<br/><br/>1) Thanks for the discussion of exact sequences, Urs. I have at times worried a lot about why one would ever be interested in "strict" exact sequences of the sort we're using here. It's nice to get a clear general picture of this, and I may add your comments - citing you, of course - to our paper. I guess whenever we construct a Lie n-algebra by extending a Lie algebra using a cocycle, we actually get a "strict" exact sequence of the sort mentioned in our paper. But yes, I see that this is a "coincidence".<br/><br/>2) Yes, we should talk about Fierz identities. We just forgot! And thanks for catching all those typos.<br/><br/>3) Urs asked:<br/><br/><blockquote><br/><br/>Can you maybe see aspects of what makes these cocycles special compared to other cocycles that the Poincaré super Lie algebra has? What other cocycles that involve the spinors are there? Maybe there are a bunch of generic cocycles and then some special ones that depend on the dimension?<br/><br/></blockquote><br/><br/>Well, of course these cocycles are special because they come from Fierz identities that hold only in certain special dimensions, and we've tried to "explain" that by giving a proof using normed division algebras. <br/><br/>But alas, we don't know reallly good answers to any of the questions you are asking here. Of course we've <i>considered</i> these questions. I think they are <i>utterly fascinating</i>. At times I've wanted John Huerta to do his thesis on these questions! But right now, other questions seem a bit easier, and perhaps interesting to more people. The questions you're asking require a highly developed expertise in representation theory.<br/><br/><blockquote><br/><br/>Is there any indication from the math to which extent (3,4,6,10) and (4,5,7,11) are the first two steps in a longer sequence of sequences? I might expect another sequence (7,8,10,14) and (11, 12, 14, 18) corresponding to the fivebrane and the ninebrane.<br/><br/></blockquote><br/><br/>If John Huerta gets really good at representation theory we could work out the full story, but right now we are mainly happy to see that the techniques we're using do <i>not</i> give a 3-brane sequence (5,6,8,12). I.e., they do not give a '5-$\Psi$'s rule' in all these dimensions. And that's what one would expect, given the apparent lack of a 3-brane theory in 12d Minkowski spacetime.<br/><br/>4) David asked:<br/><br/><blockquote><br/><br/>Aren't the first two sequences [strings in dimensions 3, 4, 6, 10 and 2-branes in dimension 4, 5, 7, 11] supposed to be from the columns in Duff's chart in the post?<br/><br/></blockquote><br/><br/>Yes, and you'll see that he <a href="http://ccdb4fs.kek.jp/cgi-bin/img/reduced_gif?198708425+15+34">explicitly links this chart to the normed division algebras</a>, but in a somewhat mysterious way, which we wanted to clarify.<br/><br/><blockquote><br/><br/>Is the specialness of 5 and 9 connected to their being (1+4) and (1+8)?<br/><br/></blockquote><br/><br/>I'm not sure what you think is special about 5 and 9. </div> -
- CommentRowNumber5.
- CommentAuthorDavid_Corfield
- CommentTimeMar 15th 2010
I was just wondering about Urs’ fivebrane and ninebrane as in the quoted portion. He then replied about the sequence (string, fivebrane, ninebrane) of dimension .
-
- CommentRowNumber6.
- CommentAuthormetaweta
- CommentTimeMar 16th 2010
The wikipedia composition algebra page says there are 1-d composition algebras when char(K) != 2. Has anyone considered your construction for char(K) != 0 or 2? -
- CommentRowNumber7.
- CommentAuthorJohn Baez
- CommentTimeMar 16th 2010
David: Okay, I get what you mean now. I think the cocycles governing Urs' fivebrane and ninebrane are "purely bosonic", unlike the ones John Huerta and I are considering. That's why I'm having trouble making any connection between what Urs is talking about now and what we did. But they should be part of the same story.
In other words: we're all interested in cocycles on the Poincaré Lie superalgebra. This superalgebra is a Z/2-graded vector space with a bracket. The even part, or "bosonic part" is an ordinary Lie algebra, namely the Lie algebra of the Poincaré group. The odd part, or "fermionic part", is the space of spinors. I think Urs is implicitly getting cocycles on the Poincaré Lie superalgebra from cocycles on its bosonic part. (Checking that this is possible requires a tiny calculation, which I am alas too busy to do right now).
What are cocycles on the Poincaré Lie algebra like? Well, it should include the cohomology of the rotation Lie algebra, and in fact that could even be all there is.
The Lie algebra of the rotation group has a bunch of interesting cocycles, related to Pontryagin classes.
If I'm not getting mixed up the Lie algebra of the rotation group has a nontrivial 3-cocycle, an nontrivial 7-cocycle, an nontrivial 11-cocycle and so on up to a certain cutoff - and if you work with rotations in high enough dimensions, this cutoff is quite high.
So, we get cocycles of degree 4k+3 for k below a certain cutoff. These give Lie (4k+2)-algebras, which in turn can be used to describe the parallel transport of (4k+1)-branes.
Oh, good - the calculation is working - it matches Urs' claim! I was worried until the end there.
But anyway, all this stuff is "purely bosonic". John Huerta and I were focusing on cocycles that only exist on the Poincaré Lie superalgebra.
I will need to think about this more someday. -
- CommentRowNumber8.
- CommentAuthorHarry Gindi
- CommentTimeMar 16th 2010
- (edited Mar 16th 2010)
Does anyone else here agree that commenting on the nForum is substantially easier (and less aggravating) than commenting on the nCafé?
-
- CommentRowNumber9.
- CommentAuthordomenico_fiorenza
- CommentTimeMar 16th 2010
me :-) (I love the cafe' for blog postings, but prefer the forum for commenting) -
- CommentRowNumber10.
- CommentAuthorUrs
- CommentTimeMar 16th 2010
John,
there are these bosonic cocycles, but I was indeed wondering about the fermionic ones.
Take the case of the string: it is governed (in the sense we are discussing here) at least by one bosonic cocycle -- the canonical 3-cocycle on
 -- and one fermionic cocycle -- the one you are discussing with John Huerta.
-- and one fermionic cocycle -- the one you are discussing with John Huerta.The super-fivebrane we know is similarly controlled by the 7-cocycle on
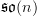 . But shouldn't there also be a fermionic cocycle to go with this, as with the string?
. But shouldn't there also be a fermionic cocycle to go with this, as with the string? -
- CommentRowNumber11.
- CommentAuthorzskoda
- CommentTimeApr 16th 2010
I created composition algebra just to have links (somebody will I hope fill some definitions and teorems).
1 to 11 of 11