nForum
Not signed in
Want to take part in these discussions? Sign in if you have an account, or apply for one below
Site Tag Cloud
Vanilla 1.1.10 is a product of Lussumo. More Information: Documentation, Community Support.
If you want to take part in these discussions either sign in now (if you have an account), apply for one now (if you don't).
- Discussion Type
- discussion topicringoid
- Category Latest Changes
- Started by Mike Shulman
- Comments 5
- Last comment by TobyBartels
- Last Active Oct 20th 2009
I think Ab-enriched category is a better name for the page than ringoid.
- Discussion Type
- discussion topic[[Jason Dusek]] joined and inspired me to start [[2-rig]].
- Category Latest Changes
- Started by TobyBartels
- Comments 1
- Last comment by TobyBartels
- Last Active Oct 20th 2009
Jason Dusek joined and inspired me to start 2-rig.
- Discussion Type
- discussion topicNew entry: [[poincare group]]
- Category Latest Changes
- Started by Todd_Trimble
- Comments 7
- Last comment by TobyBartels
- Last Active Oct 20th 2009
I wrote Poincare group as an entree to the project of carrying on in nLab the blog discussion on unitary representations of the Poincare group. I'm not a specialist of course, so I ask the experts to please examine for accuracy.
- Discussion Type
- discussion topicWrote [[short map]] and expanded [[isometry]]. (n/t)
- Category Latest Changes
- Started by TobyBartels
- Comments 1
- Last comment by TobyBartels
- Last Active Oct 20th 2009
- Discussion Type
- discussion topicG-principal oo-bundle
- Category Latest Changes
- Started by Urs
- Comments 1
- Last comment by Urs
- Last Active Oct 19th 2009
I expanded and polished the discussion of the abstract definition of of G-principal oo-bundles in an arbitrary (oo,1)-topos at principal infinity-bundle.
Parts of this could/should eventually be moved/copied to action and action groupoid, but I won't do that now.
I'd be interested in comments. One would expect that for the case that the ambient (oo,1)-topos is Top this style of definition should be well known in the literature, but I am not sure if it is. In fact, the examples listed further below in the entry, (the construction by Quillen and the Stasheff-Wirth construction) seems to indicate that this very simple very general nonsense picture has not been conceived as such before. Could that be true?
- Discussion Type
- discussion topicRequest for Help
- Category Latest Changes
- Started by Andrew Stacey
- Comments 1
- Last comment by Andrew Stacey
- Last Active Oct 19th 2009
I've removed the request for help link from the main contents. It didn't get used much (though I got answers to my questions there!). Since we have yet to actually delete a page, rather than just blank the request for help page I've put a pointer to where one can ask questions (pretty similar to that on the FAQ).
- Discussion Type
- discussion topic[[two-valued topos]]
- Category Latest Changes
- Started by TobyBartels
- Comments 3
- Last comment by Mike Shulman
- Last Active Oct 19th 2009
I wrote two-valued topos to help me tighten up Mike's latest edit to cocomplete well-pointed topos.
- Discussion Type
- discussion topicReply to Roger Witte at [[foundations]]. (n/t)
- Category Latest Changes
- Started by TobyBartels
- Comments 1
- Last comment by TobyBartels
- Last Active Oct 18th 2009
Reply to Roger Witte at foundations.
- Discussion Type
- discussion topicSch/S and S-Sch (notational point)
- Category Latest Changes
- Started by amathew
- Comments 3
- Last comment by zskoda
- Last Active Oct 18th 2009
I created a page for S-Sch as a notation for S-schemes to refer to in another post. Zoran pointed out that the notation is nonstandard (I do not know why I thought it was normal) and changed the title to Sch/S. I thus changed the first sentence to read Sch/S instead.
- Discussion Type
- discussion topicMinor changes (Hecke algebra, Hilbert basis theorem, additive envelope, etc.)
- Category Latest Changes
- Started by amathew
- Comments 4
- Last comment by JonAwbrey
- Last Active Oct 17th 2009
- I added a description of the degenerate affine Hecke algebra to the Hecke algebra page as one of the many variants.
I added the categorical generalization of Schur's lemma to that page.
I wrote a short stub on the additive envelope of a category, which Mike Shulman has expanded.
I mentioned the generalization of the Morse lemma to Hilbert manifolds.
I added the generalization of Hilbert's basis theorem to the case of where the ground ring is noetherian (not necessarily a field).
I wrote a short page on the Eilenberg swindle.
- Discussion Type
- discussion topicAkil Mathew
- Category Latest Changes
- Started by Urs
- Comments 3
- Last comment by Todd_Trimble
- Last Active Oct 17th 2009
I see that Akil Mathew has worked on a bunch of entries. Great! We should try to contact him and ask hom to record his changes here.
- Discussion Type
- discussion topicA-oo category
- Category Latest Changes
- Started by Urs
- Comments 1
- Last comment by Urs
- Last Active Oct 17th 2009
Expanded the "Idea" section at A-infinity category.
- Discussion Type
- discussion topic[[Hugh Thomas]] joined to edit [[quiver]] (n/t)
- Category Latest Changes
- Started by TobyBartels
- Comments 5
- Last comment by Urs
- Last Active Oct 16th 2009
Hugh Thomas joined to edit quiver
- Discussion Type
- discussion topicthis and that
- Category Latest Changes
- Started by Urs
- Comments 2
- Last comment by Urs
- Last Active Oct 16th 2009
I added Alex's recent lecture notes to cobordism hypothesis and in that process polished some typesetting there slightly.
Then I was pleased to note that Noah Snyder joined us and worked on fusion category. I created a page for him.
- Discussion Type
- discussion topiccocomplete well-pointed topos
- Category Latest Changes
- Started by Mike Shulman
- Comments 2
- Last comment by TobyBartels
- Last Active Oct 15th 2009
Wrote a proof at cocomplete well-pointed topos that characterizes Grothendieck universes and Set.
- Discussion Type
- discussion topicessential image
- Category Latest Changes
- Started by Mike Shulman
- Comments 1
- Last comment by Mike Shulman
- Last Active Oct 15th 2009
I don't think that the (non-full) essential image of an arbitrary functor is well-defined.
- Discussion Type
- discussion topicexamples for smooth toposes
- Category Latest Changes
- Started by Urs
- Comments 2
- Last comment by Urs
- Last Active Oct 15th 2009
I added a fairly long (but still immensely incomplete) examples section to smooth topos.
I mention the "well adapted models" and say a few words about the point of it. Then I have a sectoin on how and in which sense algebraic geometry over a field takes place in a smooth topos. here the model is described easily, but I spend some lines on how to think of this. In the last example sections I have some remarks on non-preservation of limits in included subcategories of tame objects, but all that deserves further expansion of course.
- Discussion Type
- discussion topicsmooth topos database
- Category Latest Changes
- Started by Urs
- Comments 1
- Last comment by Urs
- Last Active Oct 15th 2009
started filling in material into the planned database of smooth toposes at Models for Smooth Infinitesimal Analysis.
- Discussion Type
- discussion topicorthogonal group... in a lined topos
- Category Latest Changes
- Started by Urs
- Comments 1
- Last comment by Urs
- Last Active Oct 15th 2009
I continued working my way through the lower realms of the Whitehead tower of the orthogonal group by creating special orthogonal group and, yes, orthogonal group.
For the time being the material present there just keeps repeating the Whitehead-tower story.
But I want more there, eventually: I have a query box at orthogonal group. The most general sensible-nonsense context to talk about the orthogonal group should be any lined topos.
I am wondering if there is anything interesting to be said, from that perspective. Incidentally, I was prepared in this context to also have to create general linear group, only to find to my pleasant surprise that Zoran had already created that some time back. And in fact, Zoran discusses there an algebro-geometric perspective on GL(n) which, I think, is actually usefully thought of as the perspective of GL(n) in the lined topos of, at least, presheaves on
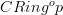 .
.Presently I feel that I want eventually a discussion of all those seemingly boring old friends such as
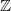 and
and  and
and 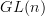 etc. in lined toposes and smooth toposes. Inspired not the least by the wealth of cool structure that even just
etc. in lined toposes and smooth toposes. Inspired not the least by the wealth of cool structure that even just 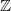 carries in cases such as the
carries in cases such as the 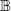 -topos in Models for Smooth Infinitesimal Analysis.
-topos in Models for Smooth Infinitesimal Analysis.
- Discussion Type
- discussion topicFivebrane group
- Category Latest Changes
- Started by Urs
- Comments 1
- Last comment by Urs
- Last Active Oct 14th 2009
created Fivebrane group but was being lazy:
essentially copy-and-pasted the intro from String group and then left a link to Fivebrane structure.
Then I went through String structure and Fivebrane structure and added links to String group and Fivebrane group.
- Discussion Type
- discussion topicHaynes Miller
- Category Latest Changes
- Started by Urs
- Comments 1
- Last comment by Urs
- Last Active Oct 14th 2009
created a page for Haynes Miller, since I just mentioned his name at string group as the one who coined that term.
not much on the page so far. Curiously, I found only a German Wikipedia page for him
- Discussion Type
- discussion topiciTeX - LaTeX differences in the FAQ
- Category Latest Changes
- Started by Andrew Stacey
- Comments 1
- Last comment by Andrew Stacey
- Last Active Oct 14th 2009
I've started listing differences between iTeX and LaTeX in the FAQ. That seemed the most logical place (I don't think we want a proliferation of places where users should look to find simple information) so either here or the HowTo seemed best. I chose the FAQ because the most likely time someone is going to look for this is when they notice something didn't look right.
The issue is that whilst iTeX is meant to be close to LaTeX they are never going to be the same so it's worth listing known differences with their work-arounds.
So far I've noted operator names, whitespace in
\text, and some oddities on number handling.
- Discussion Type
- discussion topichomotopy group (of an oo-stack)
- Category Latest Changes
- Started by Urs
- Comments 1
- Last comment by Urs
- Last Active Oct 13th 2009
created homotopy group (of an infinity-stack)
a bit rough for the time being.
Also added a suitable link and short remark at homotopy group.
- Discussion Type
- discussion topicVishal Lama
- Category Latest Changes
- Started by Urs
- Comments 1
- Last comment by Urs
- Last Active Oct 13th 2009
Vishal Lama joined the Lab!
on his page he promises to create Lab pages on some books on category theory and topos theory. Great, I am looking forward to it
- Discussion Type
- discussion topicpath-structured smoth (oo,1)-toposes
- Category Latest Changes
- Started by Urs
- Comments 3
- Last comment by Urs
- Last Active Oct 13th 2009
started working on
schreiber:path-structured (infinity,1)-toposes
This is a kind of survey of some constructions I've recently been spamming the nLab with.
- Discussion Type
- discussion topicQuestions on foundations
- Category Latest Changes
- Started by TobyBartels
- Comments 1
- Last comment by TobyBartels
- Last Active Oct 13th 2009
Roger Witte asks a question at foundations that looks interesting but which I haven't really thought about yet.
- Discussion Type
- discussion topicTodd wrote …
- Category Latest Changes
- Started by TobyBartels
- Comments 8
- Last comment by Todd_Trimble
- Last Active Oct 12th 2009
- Discussion Type
- discussion topicstructural meaning of [[axiom of foundation]]
- Category Latest Changes
- Started by TobyBartels
- Comments 1
- Last comment by TobyBartels
- Last Active Oct 12th 2009
I added a paragraph on structural meaning to axiom of foundation.
- Discussion Type
- discussion topicnLab on nLab
- Category Latest Changes
- Started by TobyBartels
- Comments 4
- Last comment by TobyBartels
- Last Active Oct 12th 2009
I added the Lab itself to Online Resources, since that list is getting a lot of attention and may well be copied to other contexts.
- Discussion Type
- discussion topicRedirection redirection
- Category Latest Changes
- Started by TobyBartels
- Comments 1
- Last comment by TobyBartels
- Last Active Oct 11th 2009
I moved the redirect for de Rham cohomology from differential form to de Rham complex.
- Discussion Type
- discussion topic[[Gram-Schmidt process]]
- Category Latest Changes
- Started by TobyBartels
- Comments 1
- Last comment by TobyBartels
- Last Active Oct 10th 2009
Todd just wrote Gram-Schmidt process; I added a bit.
- Discussion Type
- discussion topicMoved biography to people
- Category Latest Changes
- Started by TobyBartels
- Comments 2
- Last comment by Urs
- Last Active Oct 10th 2009
As planned here
- Discussion Type
- discussion topicNew pages
- Category Latest Changes
- Started by TobyBartels
- Comments 11
- Last comment by Mike Shulman
- Last Active Oct 10th 2009
pairing — pretty simple, but not to be confused with the product
- Discussion Type
- discussion topicTo go with [[simplicial category]] …
- Category Latest Changes
- Started by TobyBartels
- Comments 1
- Last comment by TobyBartels
- Last Active Oct 10th 2009
… we now have globular category.
- Discussion Type
- discussion topicsuper smooth topos
- Category Latest Changes
- Started by Urs
- Comments 5
- Last comment by TobyBartels
- Last Active Oct 10th 2009
created super smooth topos
- Discussion Type
- discussion topiccircle of entries surrounding infinitesimal neighour
- Category Latest Changes
- Started by Urs
- Comments 13
- Last comment by Eric
- Last Active Oct 9th 2009
started infinitesimal neighbour and began creating a circle of entries surrounding this:
infinitesimal path infinity-groupoid in a smooth topos; path infinity-groupoid in a smooth topos; simplex in a lined topos
This is heading in the direction of giving a full discussion of
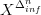 for X a microlinear space, mentioned presently already at differential forms in synthetic differential geometry. I though i could just point to the literature for that, but not quite, apparently. Anders Kock discusses this for X a "formal manifold", an object with a cover by Kock-Lawvere vector spaces. But it should work a bit more generally using microlinear spaces, as indicated in the appendix of Models for Smooth Infinitesimal Analysis. There is an obvious general-nonsense definition wich I discuss, but I need yet to insert discussion of that and how this reproduces Kock's definition (but I think it does).
for X a microlinear space, mentioned presently already at differential forms in synthetic differential geometry. I though i could just point to the literature for that, but not quite, apparently. Anders Kock discusses this for X a "formal manifold", an object with a cover by Kock-Lawvere vector spaces. But it should work a bit more generally using microlinear spaces, as indicated in the appendix of Models for Smooth Infinitesimal Analysis. There is an obvious general-nonsense definition wich I discuss, but I need yet to insert discussion of that and how this reproduces Kock's definition (but I think it does).It has been an esteemed insight for me that the best way to think of all these constructions of "combinatorial differential forms" (still have to expand the discussion of those at differential forms in synthetic differential geometry) is by organizing them into their natural simplicial structures and then noticing that the model category structure available in the background allows us to think of the resulting simplicial objects in the topos as interna oo-groupoids. I think this must clearly the nLab way of thinking about this, so I created entries with the respective titles.
You may have noticed that on my personal web I am developing the further step that goes from (infinitesimal) path oo-groupoids of objects in a 1-topos to (infinitesimal) path oo-groupoids of objects in a "smooth (oo,1)-topos". I don't want to impose that fully (oo,1)-material on the main nLab as yet, before this is further developed, but the bits now added that just have oo-groupoids of paths in a 1-topos object is straightforward enough to warrent discussion here. i think.
While working on this, I also did various minor edits on the synthetic differential geometry context cluster, such as
splitting off lined topos from smooth topos
rewriting the introduction at Models for Smooth Infinitesimal Analysis (the previous remarks are by now better explained in the corresponding sub-entries and the new summary is supposed to get the main message of the book across better). Also created section headers there for each of the single models, which I hope I'll eventually describe there in a bit more detail each. Those toposes
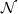 and
and  they have there are mighty cool, I think, giving not only a well-adapted model for SDG but on top of that an implementation of nonstandard analysis, and of distribution theory. I am thinking that the toposophers among my co-laborants might enjoy looking at their smooth natural number object in
they have there are mighty cool, I think, giving not only a well-adapted model for SDG but on top of that an implementation of nonstandard analysis, and of distribution theory. I am thinking that the toposophers among my co-laborants might enjoy looking at their smooth natural number object in 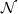 a bit more. It's fun and seems to be much more relevant than seems to be widely appreciated.
a bit more. It's fun and seems to be much more relevant than seems to be widely appreciated.Notice that at simplex in a lined topos I am asking for a reference. It's this standard construction of simplices as collpsed cylinders on lower dim simplicies. I don't think I should re-invent that wheel. What's the canonical reference for this general construction?
Finally please notice that all entries mentioned above are more or less stubby for the moment and need more work. But I thought it was about time to drop a latest-changes alert here now, before waiting longer.
- Discussion Type
- discussion topicsemiotic information
- Category Latest Changes
- Started by JonAwbrey
- Comments 1
- Last comment by JonAwbrey
- Last Active Oct 9th 2009
Inspired by David Corfield's blog entries on information geometry, I added a 'blink on semiotic information that I hope to develop over time.
- Discussion Type
- discussion topicpolsihing: infinitesimal object
- Category Latest Changes
- Started by Urs
- Comments 5
- Last comment by Urs
- Last Active Oct 9th 2009
I tried to prettify the entry infinitesimal object:
I expanded and restructured the "Idea" section. I tried to emphasize the point that Lawvere's axioms are the right general point of view and that the wealth of constructions in algebraic geoemtry is, from this abstract nonsense point of view, to be regarded as taking place in a model for these axioms. I cite Anders Kocks's latest book for the most simple minded version of how algebraic geometry is a model for sdg, but I think this goes through for more sophisticated versions, too. It would be nice to discuss this eventually elsewhere in some entry on "algebraic geometry as models for smooth toposes".
I have also tried to subsume the approach of nonsstandard analysis as yet another special case of Lawvere's general axioms, by referring to Moerdijk-Reyes' topos
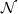 and
and  in which "objects of invertible infinitesimals/infinities" exist and model nonstandard analysis. This, too, would deserve being expanded on further, but I am thinking for the nLab this abstract-nonsense-first perspective is the right one.
in which "objects of invertible infinitesimals/infinities" exist and model nonstandard analysis. This, too, would deserve being expanded on further, but I am thinking for the nLab this abstract-nonsense-first perspective is the right one.Then I inserted some links to the now separate infinitesiaml interval object that I am still working on. I also changed the ideosyncratic terminology "infinitesimal k-cube" and "infinitsimal k-disk" to "cartesian product of inf. intervals" and "k-dimensional infinitesimal interval". Anders Kock calls the latter a "monad", following Leibniz, but I am hesitating to overload monad this way, given that Kock's use of it doesn't seem to be wide spread.
- Discussion Type
- discussion topicinfinitesimal singular simplicial object
- Category Latest Changes
- Started by Urs
- Comments 1
- Last comment by Urs
- Last Active Oct 9th 2009
Spent all day with being distracted from this single thing that I planned to finish this morning: now at least a rough sketch is done
at infinitesimal interval object in the last section with the long section name I mean to define the "infinitesimal singular simplicial complex" in a new way.
Anders Kock defines this guy for "formal manifols", roughly, for spaces that have an atlas by vector spaces. There the simple definition applies recalled at infinitesimal singular simplicial complex.
But there should be a definition for arbitrary microlinear spaces, And it should be such that it is almost manfestly the inifnitesimal version of the path oo-groupoid construction described at interval object. This is what I am aiming to describe here.
One crucial thing is that we want that morphisms out of the objects in degree k of the infinitesimal singular simplicial complex that vanish on degenerate k-simplices are automatically fiberwise skew-linear. Seeing this in the construction that I am presenting there seems to be different to the way Anders Kock describes it in the other setup. This is the main thing I need to check again when i am more awake.
- Discussion Type
- discussion topichomotopy - contents
- Category Latest Changes
- Started by Urs
- Comments 1
- Last comment by Urs
- Last Active Oct 8th 2009
I created homotopy - contents and added it as a floating table of contents to relevant entries.
This was motivated from the creation of infinitesimal interval object and the desire to provide a kind of map that shows how that concept sits in the greater scheme of things. The homotopy - contents was supposed to be a step in that diretion.
I really meant to expand at infinitesimal interval object on something I already meant to provide yesterday, but then that table of contents kept distracting me, and the fact that I came across mapping cone while editing it and felt compelled to improved that entry first, which I did
- Discussion Type
- discussion topicinterval object: induced path oo-groupoids
- Category Latest Changes
- Started by Urs
- Comments 6
- Last comment by Urs
- Last Active Oct 8th 2009
I removed my recent material at simplex in a lined topos and instead inserted this now, expanded, at
where it belongs. There is now a section there that discusses how interval objects gives rise to cubical and simplicial path oo-categories.
The proposition I state there I have carefully checked. Should be correct. But haven't typed the proof, it doesn't lend itself to being typed (straightforward but tedious, as one says).
But if it is indeed correct, this must be standard well-known stuff. Does anyone have a reference?!
I also restructured and edited the rest of the entry a bit, trying to make it a bit nicer. THis entry deserves more attention, it is a pivotal entry.
Tomorrw when I am more awake I'll remove simplex in a lined topos and redirect links to it suitably to interval oject.
- Discussion Type
- discussion topichowto and faq
- Category Latest Changes
- Started by Mike Shulman
- Comments 1
- Last comment by Mike Shulman
- Last Active Oct 8th 2009
I moved the instructions on making diagrams from FAQ to HowTo, which seemed a better fit, and added a comment about including images as another method. I also made the individual questions at FAQ into ### headers, rather than numbered lists, so that they would show up in the automatic table of contents.
- Discussion Type
- discussion topicsmooth loci
- Category Latest Changes
- Started by Urs
- Comments 2
- Last comment by TobyBartels
- Last Active Oct 8th 2009
created stub for smooth loci
(should it be "smooth locus" instead?)
- Discussion Type
- discussion topicgeneralized smooth algebra
- Category Latest Changes
- Started by Urs
- Comments 3
- Last comment by Urs
- Last Active Oct 7th 2009
sty addition to generalized smooth algebra: remark on terminology added and section on "internal definition" added.
planning to polish thinmgs later
- Discussion Type
- discussion topicsynthetic differential geometry - contents
- Category Latest Changes
- Started by Urs
- Comments 1
- Last comment by Urs
- Last Active Oct 6th 2009
started synthetic differential geometry - contents and added it as floating table of contents to the relevant entries
- Discussion Type
- discussion topicinfinitesimal space
- Category Latest Changes
- Started by Urs
- Comments 1
- Last comment by Urs
- Last Active Oct 6th 2009
quick addition of "formal infinitesimal spaces" and Weil algebras to infinitesimal space
but am planning to polish this entry further later, it is a bit of a mess at the moment
- Discussion Type
- discussion topicintegration axiom
- Category Latest Changes
- Started by Urs
- Comments 1
- Last comment by Urs
- Last Active Oct 6th 2009
started integration axiom, but incomplete for the moment
at schreiber:integration of oo-Lie algebroid valued differential forms I am thinking about the higher version of this
- Discussion Type
- discussion topiccyclic things
- Category Latest Changes
- Started by Mike Shulman
- Comments 3
- Last comment by David_Corfield
- Last Active Oct 5th 2009
Looks like maybe Todd is right at cyclic order that the cycle category cannot be defined in that way.
- Discussion Type
- discussion topicAdded detexify to online resources
- Category Latest Changes
- Started by Andrew Stacey
- Comments 1
- Last comment by Andrew Stacey
- Last Active Oct 5th 2009
Just learnt about detexify from the Secret Blogging Seminar and thought it worth adding to the online resources page.
- Discussion Type
- discussion topicNew pages
- Category Latest Changes
- Started by TobyBartels
- Comments 3
- Last comment by TobyBartels
- Last Active Oct 5th 2009
- Discussion Type
- discussion topicnatural isomorphism
- Category Latest Changes
- Started by TobyBartels
- Comments 1
- Last comment by TobyBartels
- Last Active Oct 4th 2009
A little more detail at natural isomorphism, including when one can speak of the functor satisfying certain conditions.
- Discussion Type
- discussion topichonoring Dubuc's work on synthetic differential geometry
- Category Latest Changes
- Started by Urs
- Comments 3
- Last comment by Urs
- Last Active Oct 4th 2009
By chance I came across an old CatTheory mailing list post by e. Dubuc, where he complains about how is work on SDG is not sufficiently recognized and asks people to speak of the "Dubuc topos".
I added a remark about this to synthetic differential geometry in the section on "Well adapted models".
- Discussion Type
- discussion topicI found another term for a [[tight relation]].
- Category Latest Changes
- Started by TobyBartels
- Comments 2
- Last comment by TobyBartels
- Last Active Oct 4th 2009
I found another term for a tight relation.
- Discussion Type
- discussion topicon moduli stack of elliptic curves
- Category Latest Changes
- Started by Urs
- Comments 1
- Last comment by Urs
- Last Active Oct 1st 2009
A few more sections at A Survey of Elliptic Cohomology - elliptic curves on
topol. invariants of the moduli stack of ell. curves
the compactified version
the definition of Gromov-Witten invariants
an example.
As before, this is raw material which I am thinking lends itself to be turned into entries.
- Discussion Type
- discussion topicsynthetic differential supergeometry
- Category Latest Changes
- Started by Urs
- Comments 1
- Last comment by Urs
- Last Active Oct 1st 2009
started synthetic differential supergeometry
so far just to record the (three!) references
- Discussion Type
- discussion topicpeople entries. D'Auria, Fre, Castellani, Sati
- Category Latest Changes
- Started by Urs
- Comments 1
- Last comment by Urs
- Last Active Oct 1st 2009
created entries for the following people
Riccardo D'Auria, Pietro Fre, Leonardo Castellani, Hisham Sati
not much there, yet, but these pages serve a purpose as listing the pages that link to them, which is useful I think
- Discussion Type
- discussion topicInstructions
- Category Latest Changes
- Started by TobyBartels
- Comments 5
- Last comment by TobyBartels
- Last Active Oct 1st 2009
A list of all recently edited entries can be seen at Recently Revised. But that list tends to contain lots of minor changes: it's not easy to spot the important ones. So, if you feel people's attention should be drawn to some changes you make, then please mention them here. This way the rest of us can spot them, so we can learn what you know — and maybe make further improvements!
- Go to the Latest Changes category in the Forum. If you are reading this on the forum, then you are there right now, or go to http://www.math.ntnu.no/~stacey/Vanilla/nForum/?CategoryID=5.
- Optionally, create an account (if you don't have one) and log in if necessary; this may be helpful but is not required.
- Hit ‘Start new discussion’ at the upper left.
- Choose a meaningful subject title, perhaps the name of the page you've edited or something like that.
- Write a brief message summarising your edit. Most of the formatting is the same as on the Lab if you choose the ‘Markdown+Itex’ radio button beneath the comment box.
- Please include a
[[wikilink]]to the page or pages that you edited. - If you didn't log in, then please also include your name.
- Check Preview Post (or skip it), and then Start your discussion!
Questions? Ask them here!
- Discussion Type
- discussion topicString Lie 2-algebra
- Category Latest Changes
- Started by Urs
- Comments 1
- Last comment by Urs
- Last Active Sep 30th 2009
created String Lie 2-algebra
- Discussion Type
- discussion topicexterior differential system
- Category Latest Changes
- Started by Urs
- Comments 1
- Last comment by Urs
- Last Active Sep 30th 2009
uploaded course notes by Bryant to exterior differential system (linked to from the Reference section)
- Discussion Type
- discussion topicblob homology
- Category Latest Changes
- Started by Urs
- Comments 1
- Last comment by Urs
- Last Active Sep 30th 2009
Added references to blob homology.