nForum
Not signed in
Want to take part in these discussions? Sign in if you have an account, or apply for one below
Site Tag Cloud
Vanilla 1.1.10 is a product of Lussumo. More Information: Documentation, Community Support.
If you want to take part in these discussions either sign in now (if you have an account), apply for one now (if you don't).
- Discussion Type
- discussion topic(n,1)-topos
- Category Latest Changes
- Started by Urs
- Comments 3
- Last comment by Urs
- Last Active Feb 12th 2010
stub for (n,1)-topos
- Discussion Type
- discussion topicGalois theory/bar path oo-groupoid of an oo-stack
- Category Latest Changes
- Started by Urs
- Comments 53
- Last comment by zskoda
- Last Active Feb 11th 2010
(edit: typo in the headline: meant is "bare" path oo-groupoid)
I think I have the proof that when the structured path oo-groupoid of an oo-stack oo-topos exists, as I use on my pages for differential nonabelian cohomology, then its global sections/evaluation on the point yields the bare path oo-groupoid functor, left adjoint to the formation of constant oo-stacks.
A sketch of the proof is now here.
Recall that this goes along with the discussion at locally constant infinity-stack and homotopy group of an infinity-stack.
P.S.
Am in a rush, will get back to the other discussion here that are waiting for my replies a little later. Just wanted to et this here out of the way
- Discussion Type
- discussion topicGeometric morphisms of sheaf topoi
- Category Latest Changes
- Started by TobyBartels
- Comments 3
- Last comment by TobyBartels
- Last Active Feb 9th 2010
For the record, all I did at geometric morphism#sheaftopoi was to add a paragraph at the beginning of the example, substitute ‘sober’ for ‘Hausdorff’ in appropriate places, and add to the query box there. I mention this because the diff thinks that I did much more than that, and I don't want anybody to waste time looking for such changes!
I still to make the proof apply directly to sober spaces; the part that used that the space was Hausdorff is still in those terms.
- Discussion Type
- discussion topicdirect image
- Category Latest Changes
- Started by Urs
- Comments 1
- Last comment by Urs
- Last Active Feb 9th 2010
I fiddled a bit with direct image, but maybe didn't end up doing anything of real value...
- Discussion Type
- discussion topicMenagerie
- Category Latest Changes
- Started by Tim_Porter
- Comments 8
- Last comment by Andrew Stacey
- Last Active Feb 9th 2010
I have just uploaded a new 10 chapter version of the menagerie notes. It can be got at via my n-lab page then to my personal n-lab page and follow the link.
- Discussion Type
- discussion topicSVG Editor
- Category Latest Changes
- Started by Andrew Stacey
- Comments 1
- Last comment by Andrew Stacey
- Last Active Feb 9th 2010
I've started a section in the HowTo on the new SVG-editor.
- Discussion Type
- discussion topicglobal sections
- Category Latest Changes
- Started by Urs
- Comments 4
- Last comment by Urs
- Last Active Feb 9th 2010
I expanded the Examples-section at geometric morphism and created global section to go along with constant sheaf
- Discussion Type
- discussion topic(locally) constant n-stacks
- Category Latest Changes
- Started by Urs
- Comments 8
- Last comment by Urs
- Last Active Feb 8th 2010
I started
constant infinity-stack (existed before, edited it a bit)
still need locally constant function
- Discussion Type
- discussion topiccurvature
- Category Latest Changes
- Started by zskoda
- Comments 4
- Last comment by Urs
- Last Active Feb 8th 2010
I added a new section at curvature about the classical notion of curvature and renamed the idea section into Modern generalized ideas of curvature. The classical notion has to do with bending in a space, measured in some metrics. I wrote some story about it and moved the short mention of it in previous version into that first section on classical curvature. It should be beefed up with more details. I corrected the incorrect statement in the previous version that the curvature on fiber bundles generalized the Gaussian curvature. That is not true, the Gaussian curvature is the PRODUCT of the eigenvalues of the curvature operator, rather than a 2-form. Having said that, I wrote the entry from memory and I might have introduced new errors. Please check.
- Discussion Type
- discussion topicQuestion from Todd.
- Category Latest Changes
- Started by TobyBartels
- Comments 4
- Last comment by Urs
- Last Active Feb 8th 2010
It's up high at model structure on simplicial presheaves. I think that I answered it, but Urs had probably better take a look.
- Discussion Type
- discussion topicHorns
- Category Latest Changes
- Started by Tim_Porter
- Comments 2
- Last comment by Urs
- Last Active Feb 8th 2010
Todd suggested an excellent rewording in the definition of horn, and I have made the necessary changes. Do check it out if you care about horns!
- Discussion Type
- discussion topicProfinite Homotopy Theory
- Category Latest Changes
- Started by Tim_Porter
- Comments 3
- Last comment by Tim_Porter
- Last Active Feb 8th 2010
I noted the point made by, I think, Toby about there being stuff on profinite homotopy type in the wrong place (profinite group). I have started up a new entry on profinite homotopy types, but am feeling that it needs some more input of ideas, so help please.
- Discussion Type
- discussion topiccohomology
- Category Latest Changes
- Started by Urs
- Comments 1
- Last comment by Urs
- Last Active Feb 5th 2010
added a paragraph on Lurie's HTT section 7.2.2 to References at cohomology
- Discussion Type
- discussion topicVocabulary words from Stone Spaces, II.1.3
- Category Latest Changes
- Started by TobyBartels
- Comments 1
- Last comment by TobyBartels
- Last Active Feb 5th 2010
- Discussion Type
- discussion topicfundamental group of a topos
- Category Latest Changes
- Started by Urs
- Comments 1
- Last comment by Urs
- Last Active Feb 5th 2010
I edited fundamental group of a topos a bit
added more subsections, trying to make the structure of the entry clearer
added an Idea-in-words paragraph at the very beginning, before Tim Portert's idea-of-the-technical-construction part now following this.
where the discussion alluded to higher toposes I have now linked to the relevant entry fundamental group of an infinity-stack
added the reference to Johnstone's book
added at homotopy group of an infinity-stack links back to this entry here, as well as at Grothendieck's Galois theory.
- Discussion Type
- discussion topicn-Hom-categories
- Category Latest Changes
- Started by TobyBartels
- Comments 6
- Last comment by TobyBartels
- Last Active Feb 5th 2010
These are used by Sridhar Ramesh to great expository effect at (n,r)-category.
- Discussion Type
- discussion topicgauge fixing
- Category Latest Changes
- Started by domenico_fiorenza
- Comments 11
- Last comment by TobyBartels
- Last Active Feb 4th 2010
- I'd like to create an entry gauge fixing. the usual method I use to create new entries (typing their name in the search form) does not work, since search redirects me to examples for Lagrangian BV. now I'm trying to put a link here to see if this works (but I'm skeptic..). should it not work, how do I create the new entry?
thanks
edit: it works!!!! :-)
I'll now start writing the entry
- Discussion Type
- discussion topiclocal system
- Category Latest Changes
- Started by Urs
- Comments 1
- Last comment by Urs
- Last Active Feb 4th 2010
I am a bit unhappy with the present state of local system. This entry is lacking the good systematic nPOV story that would hold it together.
(For instance at some point a local system is defined to be a locally constant sheaf with values in vector spaces . This is something a secret blogger would do, but not worthy of an nLab. Certainly that's in practice an important specia case, but still just a very special case).
But I don*t just want to restructure the entry without getting some feedback first. So I added now at the very end a section
To my mind this should become, with due comments not to scare the 0-category theorists away, the second section after a short and to-the-point Idea section. The current Idea section is too long (I guess I wrote it! :-)
Give me some feedback please. If I see essential agreement, I will take care and polish the entry a bit, accordingly.
- Discussion Type
- discussion topicRepresentations and intertwiners
- Category Latest Changes
- Started by TobyBartels
- Comments 12
- Last comment by Urs
- Last Active Feb 4th 2010
We've had a stub at representation for awhile; I rewrote it and let intertwiner redirect to it. But it's still a stub.
- Discussion Type
- discussion topicmaterial-structural adjunction
- Category Latest Changes
- Started by Mike Shulman
- Comments 2
- Last comment by TobyBartels
- Last Active Feb 3rd 2010
Created material-structural adjunction, along with a stub for Mostowski's collapsing lemma.
- Discussion Type
- discussion topicSize issues
- Category Latest Changes
- Started by TobyBartels
- Comments 4
- Last comment by TobyBartels
- Last Active Feb 2nd 2010
I would like to write an article at size issues about the various ways of dealing with them, and I've started linking to that page in anticipation.
I still haven't written it, but I decided that there were enough links that I ought to put something. So now there are some links to other articles in the Lab on the subject.
- Discussion Type
- discussion topicCodifferential
- Category Latest Changes
- Started by Tim_Porter
- Comments 4
- Last comment by zskoda
- Last Active Feb 2nd 2010
- I noticed the word codifferential was used in the page on chain complexes. I raised this sort of terminological problem before and cannot remember the result of the discussion! The boundary operator in a chain complex is classically called the `differential', and the extra co seems contrary to `tradition'. Perhaps cochain complexes should have a cofferential but even that seems unnecessary. I have not changed this in case someone else has a good reason for the terminology ... what is the concensus? The point is not important but is worth clearing up I think.
- Discussion Type
- discussion topicEssential suprema
- Category Latest Changes
- Started by TobyBartels
- Comments 1
- Last comment by TobyBartels
- Last Active Feb 2nd 2010
Somebody clicked some buttons to make an empty slideshow at essential supremum, so I wrote an extremely stubby article there with links to real articles on the rest of the WWW.
- Discussion Type
- discussion topicCoo-ring
- Category Latest Changes
- Started by Urs
- Comments 1
- Last comment by Urs
- Last Active Feb 2nd 2010
Expanded the References section at Coo-rings
(in the course of creating Seminar on Smooth Loci (schreiber))
came across one more Coo-ring reference by Peter Michor: on characterizong those Coo-rings that are algebras of smooth functions on some manifold. Interesting.
- Discussion Type
- discussion topicsSet-categories and quasi-categories
- Category Latest Changes
- Started by Urs
- Comments 13
- Last comment by Tim_Porter
- Last Active Feb 2nd 2010
I want to eventually expand the stub entry relation between quasi-categories and simplicial categories. I just added a sentence only to give the entry something like an "Idea" section wher previously there had been just a lonely hyperlink.
I think the main theme here is that of "semi-strictification". So I added a remark to semi-strict infinity-category. But not really anything satisfactory yet.
- Discussion Type
- discussion topic(Regular) subframes
- Category Latest Changes
- Started by TobyBartels
- Comments 1
- Last comment by TobyBartels
- Last Active Feb 2nd 2010
I started subframe; of course, a subframe corresponds to a quotient locale.
But we really want regular subframes. Is there a convenient elementary description of those?
- Discussion Type
- discussion topic(oo,1)-category theory
- Category Latest Changes
- Started by Urs
- Comments 1
- Last comment by Urs
- Last Active Feb 2nd 2010
I felt that we needed an explicit entry
to collect the notions of category theory available in the context of (oo,1)-categories.
I had first started making this a subsection at (infinity,1)-category, but then I felt that this should parallel category and category theory.
In this context I then also felt like creating (infinity,1)-Grothendieck construction. This so far is just a collection of pointers, but eventually for instance some of the material currently hidden at limit in a quasi-category should be moved here.
- Discussion Type
- discussion topicidempotent adjunction
- Category Latest Changes
- Started by Mike Shulman
- Comments 9
- Last comment by zskoda
- Last Active Feb 1st 2010
Created idempotent adjunction. Does anyone know a published reference for this notion?
- Discussion Type
- discussion topicDirected joins
- Category Latest Changes
- Started by TobyBartels
- Comments 2
- Last comment by TobyBartels
- Last Active Feb 1st 2010
A little article: directed join
- Discussion Type
- discussion topicultrafilters
- Category Latest Changes
- Started by David_Corfield
- Comments 1
- Last comment by David_Corfield
- Last Active Feb 1st 2010
- Found some exposition by Todd on the web to add to ultrafilter, and a quote by Michal Barr added at ultraproduct.
This was prompted by a comment by Terry Tao
>There are two main facts that makes ultralimit analysis powerful. The first is that one can take ultralimits of arbitrary sequences of objects, as opposed to more traditional tools such as metric completions, which only allow one to take limits of Cauchy sequences of objects.
So are ultralimits in his sense a form of completion?
- Discussion Type
- discussion topicquestions on structured (infty,1)-topoi
- Category Latest Changes
- Started by Mike Shulman
- Comments 57
- Last comment by Urs
- Last Active Feb 1st 2010
Several naive questions at structured (infinity,1)-topos.
- Discussion Type
- discussion topicOpen maps
- Category Latest Changes
- Started by TobyBartels
- Comments 1
- Last comment by TobyBartels
- Last Active Jan 31st 2010
Definitions for topological spaces, locales, and toposes here: open map.
- Discussion Type
- discussion topicNew article: CABAs
- Category Latest Changes
- Started by TobyBartels
- Comments 6
- Last comment by TobyBartels
- Last Active Jan 31st 2010
Does anybody know of a definition of CABA (which would not literally be complete atomic Boolean algebra) such that the theorem that the CABAs are (up to isomorphism of posets) precisely the power sets is constructively valid?
If you do, you can put it here: CABA.
- Discussion Type
- discussion topicPreservation of limits
- Category Latest Changes
- Started by TobyBartels
- Comments 3
- Last comment by TobyBartels
- Last Active Jan 31st 2010
I created a page on this topic: preserved limit. (We also need reflected limit and created limit.)
I'm not sure about the stuff at the bottom.
- Discussion Type
- discussion topiccohomology
- Category Latest Changes
- Started by Urs
- Comments 13
- Last comment by Mike Shulman
- Last Active Jan 30th 2010
I further worked on the Idea-section at cohomology, expanding and polishing here and there.
Hit "see changes" to see what I did, precisely: changes (additions, mostly) concern mainly the part on nonabelian cohomology, and then at the end the part about twisted and differential cohomology.
by the way, what's your all opinion about that big inset by Jim Stasheff following the Idea section, ended by Toby's query box? I think we should remove this.
This is really essentially something I once wrote on my private web. Jim added some sentences to the first paragraph. Possibly he even meant to add this to my private web and by accident put it on the main Lab. In any case, that part is not realy fitting well with the flow of the entry (which needs improvement in itself) and most of the information is repeated anyway. I am pretty sure Jim won't mind if we essentially remove this. We could keep a paragraph that amplifies the situation in Top a bit, in an Examples-section.
What do you think?
- Discussion Type
- discussion topicon structured (oo,1)-topoi
- Category Latest Changes
- Started by Urs
- Comments 11
- Last comment by zskoda
- Last Active Jan 29th 2010
<p>Since Mike's thread <a href="http://www.math.ntnu.no/~stacey/Vanilla/nForum/comments.php?DiscussionID=679&page=1">questions on structured (oo,1)-topos</a> got a bit highjacked by general oo-stack questions, I thought I'd start this new thread to announce attempted answers:</p> <p>Mike had rightly complained in a query box that a "remark" of mine in which I had meant to indicate the intuitive meaning of the technical condition on an (oo,1)-structure sheaf had been "ridiculous".</p> <p>But that condition is important, and important to understand. I have now removed the nonsensical paragraph and Mike's query box complaining about it, inserted a new query box saying "second attempt" and then spelled out two archetypical toy examples in detail, that illustrate what's going on.</p> <p>The second of them can be found in StrucSp itself, as indicated. It serves mainly to show that an ordinary ringed space has a structure sheaf in the sense of structured oo-toposes precisely if it is a <a href="https://ncatlab.org/nlab/show/locally+ringed+space">locally ringed space</a>.</p> <p>But to try to bring out the very simple geometry behind this even better, I preceded this example now by one where a structure sheaf just of continuous functions is considered.</p> <p>Have a look.</p>
- Discussion Type
- discussion topiccosimplicial simplicial abelian groups and rings
- Category Latest Changes
- Started by Urs
- Comments 3
- Last comment by Urs
- Last Active Jan 28th 2010
can anyone point me to some useful discussion of cosismplicial simplicial abelian groups
![\Delta \to [\Delta^{op}, Ab] \Delta \to [\Delta^{op}, Ab]](/extensions/vLaTeX/cache/latex_d1cc8ee3ed1bc3649a9309085cdbd8e6.png)
and cosimplicial simplicial rings
![\Delta \to [\Delta^{op}, CRing] \Delta \to [\Delta^{op}, CRing]](/extensions/vLaTeX/cache/latex_5d63c4f0c914aa3a558311cfc687015e.png)
I guess there should be a Dold-Kan correspondence relating these to unbounded (co)chain complexes (that may be nontrivial both in positive as well as in negative degree). I suppose it's kind of straightforward how this should work, but I'd still ike to know of any literature that might discuss this. Anything?
- Discussion Type
- discussion topicbigrading on cohomology
- Category Latest Changes
- Started by Urs
- Comments 1
- Last comment by Urs
- Last Active Jan 28th 2010
I expanded the section Gradings at cohomology. Made three sub-paragraphs:
integer grading
bigrading
exoctic grading
using the kind of insights that we were discussing recently in various places.
I also put in a query box where I wonder about a nice way to define a Chern character construction for general oo-stack oo-toposes.
- Discussion Type
- discussion topicCoo-rings
- Category Latest Changes
- Started by Urs
- Comments 4
- Last comment by zskoda
- Last Active Jan 27th 2010
added an interesting reference by Kriegl and Michor to generalized smooth algebra, kindly pointed out by Thomas Nikolaus:
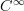 -algebras from the functional analyytic point of view
-algebras from the functional analyytic point of viewAlso added some other references.
- Discussion Type
- discussion topicBrown's Abstract Homotopy Theory and Generalized Sheaf Cohomology
- Category Latest Changes
- Started by Urs
- Comments 1
- Last comment by Urs
- Last Active Jan 25th 2010
I completely rewrote BrownAHT
same message as before, but I think I could say it much better now
- Discussion Type
- discussion topicde Rham space as derived free loop space
- Category Latest Changes
- Started by Urs
- Comments 1
- Last comment by Urs
- Last Active Jan 24th 2010
added a quick mention of the relation between deRham space and derived free loop space object to derived stack and Hochschild cohomology, pointing to this reference here for all the details
B. Toen, G. Vezzosi,
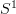 -Equivariant simplicial algebras and de Rham theory http://arxiv.org/abs/0904.3256
-Equivariant simplicial algebras and de Rham theory http://arxiv.org/abs/0904.3256more later.
- Discussion Type
- discussion topicrelative cohomology
- Category Latest Changes
- Started by Urs
- Comments 9
- Last comment by TobyBartels
- Last Active Jan 23rd 2010
this MO question on relative cohomology made me create an entry with an attempt to give the fully general abstract definition in the spirit of the discussion at cohomology
a very stubby note is now at relative cohomology
this clearly needs more development, but I think the basic idea is obvious
- Discussion Type
- discussion topicmotivic cohomology as stable oo-stack cohomology
- Category Latest Changes
- Started by Urs
- Comments 12
- Last comment by Urs
- Last Active Jan 21st 2010
I added to motivic cohomology the definition of motivic cohomology in terms of connected components of hom spaces in (the homotopy stabilization of) the (oo,1)-topos of oo-stacks on the Nisnevich site.
This is now the section Homotopy localization of the (oo,1)-topos on Nis.
Probably Zoran mentioned this before, without me getting it. Now I get it, and I like it a lot. This is the kind of nice formulation of motivic cohomology that one would expect from the point of view that is expressed at cohomology:
in fact, really a priori one should consider nonabelian motivic cohomology, by considering genuine oo-stacks on Nis, without stabilizing. One could consider differential Motivic cohomology by not retricting attention to the homotopy localization .
- Discussion Type
- discussion topichypersimplex
- Category Latest Changes
- Started by zskoda
- Comments 2
- Last comment by zskoda
- Last Active Jan 21st 2010
I entered hypersimplex, which is a tip of an important iceberg in stable category business, I hope we can continue expanding the topic and do osme research on it.
- Discussion Type
- discussion topicinterval object: dendroidal version of path groupoid
- Category Latest Changes
- Started by Urs
- Comments 1
- Last comment by Urs
- Last Active Jan 19th 2010
at interval object we have a section that discusses how in a category with interval object for every object there are various incarnations of its "path groupoid".
We had had two such incarnations there: the first one discusses the structure of a Trimble n-category on this "path groupoid", the second one the structure of a simplicial set.
I want one more such incarnation: the structure of a planar dendroidal set.
A proposal for how that should work I have now typed in the new section titled currently Fundamental little 1-cubes space induced from an interval.
(This section title is bad, I need to think of something better...)
Eventually I want to see if this can be pushed to constitute the necessary ingredients for a "May recognition principle" in a general oo-stack oo-topos: over a site C with interval object, I want for each k a dendroidal presheaf that encodes something like the C-parameterized little k-cubes operad, which should act on k-fold loop oo-stacks on C.
That's the motivation, at least.
- Discussion Type
- discussion topicquasicoherent oo-stack
- Category Latest Changes
- Started by Urs
- Comments 10
- Last comment by Urs
- Last Active Jan 18th 2010
I started quasicoherent infinity-stack. Currently all this contains is a summary of some central definitions and propositions in Toen/Vezzosi's work. I tried to list lots of direct pointers to page and verse, as their two articles tend to be a bit baroque as far as notation and terminology is concerned.
This goes parallel with the blog discussion here.
In the process I also created stubs for SSet-site and model site. These are terms by Toen/Vezzosi, but I think these are obvious enough concepts that deserve an entry of their own. Eventually we should also have one titled "(oo,1)-site", probably, that points to these as special models.
- Discussion Type
- discussion topicsmooth natural numbers
- Category Latest Changes
- Started by Urs
- Comments 27
- Last comment by Urs
- Last Active Jan 18th 2010
created an entry smooth natural numbers
I tried to extract there the fundamental mechanism that makes the "nonstandard natural numbers" in Moerdijk-Reyes Models for Smooth Infinitesimal Analysis tick. In their book the basic idea is a bit hidden, but in fact it seems that it is a very elementary mechanism at work. I try to describe that at the entry. Would be grateful for a sanity check from topos experts.
I find it pretty neat how the sequences of numbers used to represent infinite numbers in nonstandard analysis appears (as far as I understand) as generalized elements of a sheaf in a sheaf topos here.
- Discussion Type
- discussion topicFredholm operator and K-theory
- Category Latest Changes
- Started by Urs
- Comments 1
- Last comment by Urs
- Last Active Jan 15th 2010
I noticed that we have an entry Fredholm operator. I added a very brief remark on the space of Fredholm operators as a classifying space for topological K-theory , and added there a very brief link back.
eventually, of course, it would be nice to add some details.
(also added sections and a toc).
- Discussion Type
- discussion topiclecture notes
- Category Latest Changes
- Started by Urs
- Comments 6
- Last comment by Urs
- Last Active Jan 15th 2010
I once again can't enter the edit pages. So this here is just to remind myself:
I just discovered that the lecture notes for the Barcelona school a while ago are in fact online available, here:
Advanced Course on Simplicial Methods in Higher Categories
This should have been out as a book already, but keeps being delayed. It contains three important lectures, that we should link to from the respective entries:
Joyal's book on quasicategories
Moerdijk's book on dendroidal sets
Toen's lectures on simplicial presheaves
- Discussion Type
- discussion topicCategory Theory Problem on Right Adjoints
- Category Latest Changes
- Started by yellsap
- Comments 3
- Last comment by TobyBartels
- Last Active Jan 15th 2010
- How can I upload a document with diagrams that people can view the problem.
- Discussion Type
- discussion topicNew page: [[relative point of view]]
- Category Latest Changes
- Started by TobyBartels
- Comments 4
- Last comment by zskoda
- Last Active Jan 14th 2010
I started relative point of view based on material in the pages that link to it.
- Discussion Type
- discussion topicweighted join of quasi-categories
- Category Latest Changes
- Started by domenico_fiorenza
- Comments 12
- Last comment by Mike Shulman
- Last Active Jan 14th 2010
- in order to discuss weighted limits in my revision of limits, I introduced a stubby notion of weighted join of quasi-categories. The construction and the subsequent notion of weighted limit seem quite natural, but everithing now seems too simple, so I fear to have completely misunderstood the notion of weighted limit.. :(
could anybody give a look?
- Discussion Type
- discussion topicSullivan differential forms
- Category Latest Changes
- Started by Urs
- Comments 1
- Last comment by Urs
- Last Active Jan 13th 2010
- Discussion Type
- discussion topiccocycle
- Category Latest Changes
- Started by Urs
- Comments 2
- Last comment by Urs
- Last Active Jan 11th 2010
started an entry cocycle to go along with the entry cohomology, motivated from my discussion with Mike on the blog here
I mention the possible terminology suggeestion of "anamorphisms" for cocycles there, and added a link to it from anafunctor.
- Discussion Type
- discussion topicWhitehead tower
- Category Latest Changes
- Started by Guest
- Comments 19
- Last comment by domenico_fiorenza
- Last Active Jan 10th 2010
- started Whitehead tower, plus some speculative comments on versions using higher categories.
-David Roberts
- Discussion Type
- discussion topicinternal oo-groupoid
- Category Latest Changes
- Started by Urs
- Comments 4
- Last comment by domenico_fiorenza
- Last Active Jan 9th 2010
I found the discusssion at internal infinity-groupoid was missing some perspectives
I made the material originally there into one subsection called
- Kan complexes in an ordinary category
and added two more subsections
Kan complexes in an (oo,1)-category
Internal strict oo-groupoids .
The first of the two currently just points to the other relevant entry, which is groupoid object in an (infinity,1)-category, the second one is currently empty.
But I also added a few paragraphs in an Idea section preceeding everything, that is supposed to indicate how things fit together.
- Discussion Type
- discussion topic(infinity,2)-category
- Category Latest Changes
- Started by Urs
- Comments 1
- Last comment by Urs
- Last Active Jan 8th 2010
added to (infinity,2)-category a section models for the (oo,1)-category of all (oo,2)-categories
I also added (infinity,2)-category and Theta-space to the floating TOC
- Discussion Type
- discussion topic2-fibration at codomain fibration
- Category Latest Changes
- Started by Guest
- Comments 3
- Last comment by zskoda
- Last Active Jan 8th 2010
- Comment at codomain fibration about the suggested categorification, Cat^2 --> Cat. I personally don't think we've got to the bottom of what a 2-fibration is, with the possible exception of Igor Bakovic.
David Roberts
- Discussion Type
- discussion topicEilenberg–Mac Lane shenanigans
- Category Latest Changes
- Started by TobyBartels
- Comments 3
- Last comment by Urs
- Last Active Jan 8th 2010
I've just discovered that, from back in the days before redirects, we have two versions of Eilenberg-Mac Lane space. I have now combined them, by brute force; I'll leave it to Urs to make it look nice.
- Discussion Type
- discussion topicuniray group
- Category Latest Changes
- Started by Urs
- Comments 9
- Last comment by zskoda
- Last Active Jan 7th 2010
stubby stub for unitary group